1. Tardigrade
  2. Question
  3. Mathematics
  4. For the given LPP (Linear Programming Problem) max z=5x+3y 2x+y le 12 3x+2y le 20 x ge 0, y ge 0 the optimal solution set is

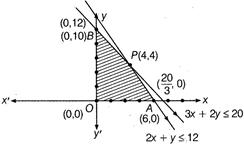
Q. For the given LPP (Linear Programming Problem) max $ z=5x+3y $ $ 2x+y\le 12 $ $ 3x+2y\le 20 $ $ x\ge 0,\,y\ge 0 $ the optimal solution set is

J & K CETJ & K CET 2013Linear Programming Report Error

Solution:

Given LPP is $ Max\,z=5x+3y $ $ 2x+y\le 12 $ $ 3x+2y\le 20 $ $ x\ge 0,\,\,\,\,\,\,\,\,\,y\ge 0 $ First we consider all the inequalities as equations.
Equations
Points
$ 2x+y=12 $
$ (0,\,\,12),\,\,(6,0) $
and
$ (0,\,\,10),\,\,\,\,\left(
$ 3x+2y=20 $
\frac{20}{3},0 \right) $
Now, plot all these points on a graph paper and make a figure.
For intersection point P, solve both equation of lines, we get $ \begin{align} & 4x+2y=24 \\ & 3x+2y=20 \\ & -\,\,\,\,\,\,\,\,\,\,-\,\,\,\,\,\,\,\,\,- \\ & \_\_\_\_\_\_\_\_\_\_ \\ & -5x=-6 \\ \end{align} $ $ \Rightarrow $ $ x=\frac{6}{5} $ and then $ y=\frac{22}{5} $ $ \therefore $ Convex region is TSQD with extreme point $ T(0,8),\,\,S(1,5),\,Q(2,4) $ and $ D(10,0) $ . Now, apply corner point method
Points
Objective function Max $ Z=5x+3y $
$ O(0,0) $
$ 5\times 0+3\times 0=0 $
$ B(0,10) $
$ 5\times 0+3\times 10=30 $
$ P(4,4) $
$ 5\times 4+3\times 4=32(\max ) $
$ A(6,0) $
$ 5\times 6+3\times 0=30 $
$ \therefore $ Optimal solution set is $ (4,4) $ on which the objective function is maximize.

Solution Image

Questions from J & K CET 2013

1. A survey shows that $63\%$ of the Americans like cheese where as $76\%$ like apples. If $x\%$ of the Americans like both cheese and apples, then the value of $x$ is

Sets

2. The value of $ \cos \left( \frac{3\pi }{2}+x \right)\,\cos \,(2\pi +x)\left\{ \cot \left( \frac{3\pi }{2}-x \right)+\cot \,(2\pi +x) \right\} $ is

Trigonometric Functions

3. If $ \sin B=3\sin (2A+B), $ then $ 2\tan A+\tan (A+B) $ =

Trigonometric Functions

4. If $ \theta =\frac{\pi }{{{2}^{n}}+1}, $ then the value of $ {{2}^{n}}\cos \theta \,\cos \,2\theta \,\cos \,{{2}^{2}}\theta .....\cos {{2}^{n-1}}\theta $ is

Trigonometric Functions

5. Using the principal values, the value of $ {{\sin }^{-1}}\left\{ \sin \frac{5\pi }{6} \right\}+{{\tan }^{-1}}\left\{ \tan \frac{\pi }{6} \right\} $ = ?

Inverse Trigonometric Functions

Questions from Linear Programming

1. The objective function $z = x_{1} + x_{2}$, subject to $x_{1} + x_{2} ≤ 10, - 2x_{1} + 3x_{2} ≤ 15, x_{1} ≤ 6, x_{1} , x_{2} ≥ 0$ has maximum value ______________ of the feasible region.

Maharashtra CET 2016

2. For the given LPP (Linear Programming Problem) max $ z=5x+3y $ $ 2x+y\le 12 $ $ 3x+2y\le 20 $ $ x\ge 0,\,y\ge 0 $ the optimal solution set is

J & K CET 2013

3. Consider the Linear Programming Problem Maximum
$ Z = 4x + y $
Subject to $ x + y \le 50 $
$ x + y \ge 100 $
$ x,y \ge 0 $
The maximum value of $ Z $ is

AMU 2012

4. The maximum value of $ Z = 4x + y $ subject to the constraints, $ x + y \le,50, 3x+y \le 90, x\ge 0, y \ge 0 $ is

AMU 2010

5. A dietician wishes to mix together two kinds of food $X$ and $Y$ in such a way that the mixture contains atleast $10$ units of vitamin $A, 12$ units of vitamin $B$ and $8$ units of vitamin $C$. The vitamin content of $1\, kg$ food is given below

J & K CET 2013

Mathematics Most Viewed Questions

1. The solution of $\frac{dy}{dx} = \frac{y}{x}+\tan \frac{y}{x}$ is

WBJEE 2011 Differential Equations

2. The solution of the differential equation $\frac{dy}{dx} = (x +y)^2$ is

COMEDK 2009 Differential Equations

3. If a and b are vectors such that $|a+b|=|a-b|$ then the angle between a and b is

KCET 2007 Vector Algebra

4. If $\begin{bmatrix}1&- \tan\theta \\ \tan \theta&1\end{bmatrix}\begin{bmatrix}1&\tan \theta \\ - \tan \theta &1\end{bmatrix}^{-1} = \begin{bmatrix}a&-b\\ b&a\end{bmatrix}$ then

COMEDK 2009 Matrices

5. $\int\frac{1}{\sin x\, \cos x}$ dx is equal to

KEAM 2016 Integrals

6. $\int\frac{\sin \frac{5x}{2}}{\sin \frac{x}{2}} dx $ is equal to :
(where $c$ is a constant of integration)

JEE Main 2019 Integrals

7. A particle is dropped under gravity from rest from a height $ h(g=9.8\,m/{{s}^{2}}) $ and it travels a distance $ \frac{9h}{25} $ in the last second the height $ h $ is:

Bihar CECE 2006

8. The value of $ \int{\frac{{{x}^{2}}+1}{{{x}^{4}}-{{x}^{2}}+1}}dx $ is

KEAM 2007 Integrals

9. The value of $\int \frac{x^2+1}{x^2-1}dx$ is

KCET 2007 Integrals

10. The value of the integral $\int\frac{cos x}{sin x + cos x}dx$ is equal to

KEAM 2013 Integrals

Latest Updates