JEE Main Question Paper with Solution 2022 July 25th Shift 1 - Morning

A

$\left[ P A ^{-1} T ^0\right]$

B

$\left[ P A T ^{-1}\right]$

C

$\left[ PA ^{-1} T \right]$

D

$\left[ P A ^{-1} T ^{-1}\right]$

Solution

Viscosity $=$ pascal.second
$P ^{ x } A ^{ y } T ^{ z }=\left[ M ^1 L ^{-1} T ^{-1}\right]$
${\left[ M ^1 L ^{+1} T ^{-1}\right]^{ x }\left[ L ^2\right]^{ y }\left[ T ^1\right]^{ z }= M ^1 L ^{-1} T ^{-1}}$
$M ^{ x } L ^{+ x +2 y } T ^{- x + z }= M ^1 L ^{-1} T ^{-1}$
$x =1 \,\, x +2 y =-1 \,\,- x + z =-1$
$y =-1$
$z =0$
Viscosity $= P ^1 A ^{-1} T ^0$

A

Electric displacement $(\vec{D})$ and surface charge density

B

Displacement current and electric field

C

Current density and surface charge density

D

Electric potential and energy

Solution

Electric displacement
$\vec{ D }=\epsilon_0 \vec{ E }$
${[ D ]=\left[\epsilon_0 E \right]=\left[\epsilon_0 \frac{\sigma}{\epsilon_0}\right]}$
${[ D ]=[\sigma]}$
$\rightarrow$ Surface change density $=\sigma$.

A

42 m

B

47 m

C

19 m

D

40 m

Solution

$d = R \theta$
$60= R \left(\frac{3 \pi}{4}\right)$
$R =\frac{60 \times 4}{3 \pi}=\frac{80}{\pi} m$
Displacement $=\sqrt{ R ^2+ R ^2-2 R ^2 \cos 135}$
$\Rightarrow \sqrt{2 R ^2-2 R ^2(-0.7)}$
$\Rightarrow \sqrt{3.4 R ^2}=\sqrt{3.4\left(\frac{80}{\pi}\right)^2}$
$\approx 47 m$

A

64 J

B

60 J

C

120 J

D

128 J

Solution

$v _{ i }=3\left(0^2\right)+4=4 \cong x =0$
$v _{ F }=3(2)^2 \mid 4 \cong x =2$
$=16$
$W =\Delta K =\frac{1}{2} m \left(16^2-4^2\right)$
$=\frac{1}{2} \times \frac{1}{2}(256-16)$
$=\frac{240}{4}=60 \,J$

A

$\sqrt{\frac{5}{3}}$

B

$\sqrt{\frac{4}{5}}$

C

$\sqrt{\frac{3}{5}}$

D

$\sqrt{\frac{14}{15}}$

Solution

$V =\sqrt{\frac{2 gH }{1+ k ^2 / R ^2}}$
$\frac{ V _{\text {cylinder }}}{ V _{\text {sphere }}}=\sqrt{\frac{\left(1+ k ^2 / R ^2\right)_{\text {sphere }}}{\left(1+ k ^2 / R ^2\right)_{\text {cylinder }}}}$
$=\sqrt{\frac{1+2 / 5}{1+1 / 2}}=\sqrt{\frac{7}{5} \times \frac{2}{3}}=\sqrt{\frac{14}{15}}$

A

21 G

B

100 G

C

59 G

D

42 G

Solution

$F =\frac{ GMM }{ r ^2}+\sqrt{2} \frac{ GMM }{(\sqrt{2} r )^2}$
$=\frac{ GMM }{ r ^2}\left(1+\frac{1}{\sqrt{2}}\right)$
$=\frac{ G \times 10^4}{13^2}\left(1+\frac{1}{\sqrt{2}}\right)$
$F \simeq 100 G$

A

$3.536 \times 10^5 Pa$

B

$3.536 \times 10^6 Pa$

C

$1.25 \times 10^6 Pa$

D

$1.25 \times 10^5 Pa$

Solution

$P _1=2 \times 10^7 Pa$
$P _1 V _1= P _2 V _2$
Since $V_2=2 V_1$ Hence $P_2=P_1 / 2$ (isothermal expansion)
$P_2=1 \times 10^7 Pa$
$P_2\left(V_2\right)^\gamma=P_3\left(2 V_2\right)^\gamma$
$P_3=\frac{1 \times 10^7}{2^{1.5}}=3.536 \times 10^6$

A

(1) and (4) only

B

(1), (2) and (4) only

C

(2) and (4) only

D

(1), (2) and (5) only

Solution

$KE _{\text {avg }}=\frac{3}{2} KT$
$P =\frac{1}{3} \rho V _{ rms }^2$
Note : Statement (4) is correct only if we consider it at constant volume and not constant pressure. Ideally, this question must be bonus but most appropriate answer is option (A)

A

$\frac{T_1}{T_2}=\frac{3}{\sqrt{2}}$

B

$\frac{T_1}{T_2}=\sqrt{\frac{3}{2}}$

C

$\frac{ T _1}{ T _2}=\sqrt{\frac{2}{3}}$

D

$\frac{ T _1}{ T _2}=\frac{\sqrt{2}}{3}$

Solution

$T _1=2 \pi \sqrt{\frac{3 m }{2 k }}$
$T _2=2 \pi \sqrt{\frac{ m }{3 k }}$
$\frac{ T _1}{ T _2}=\frac{2 \pi \sqrt{\frac{3 m }{2 k }}}{2 \pi \sqrt{\frac{ m }{3 k }}}=\frac{3}{\sqrt{2}}$

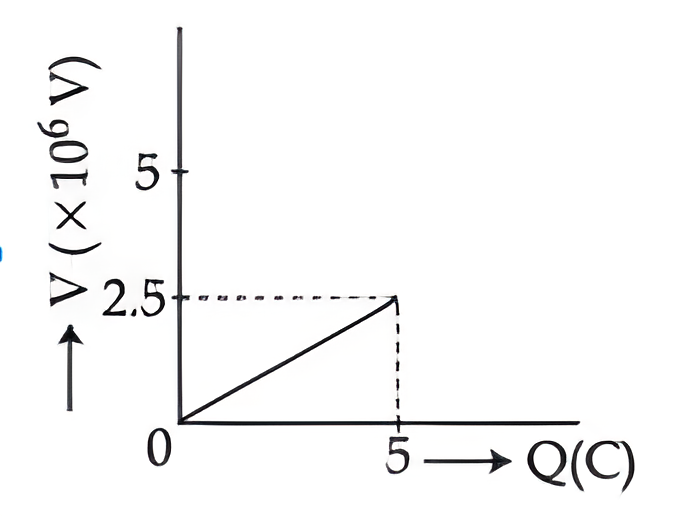
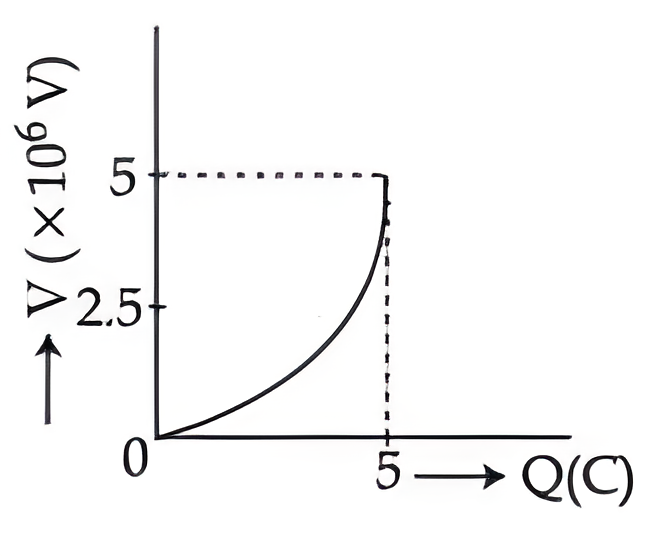
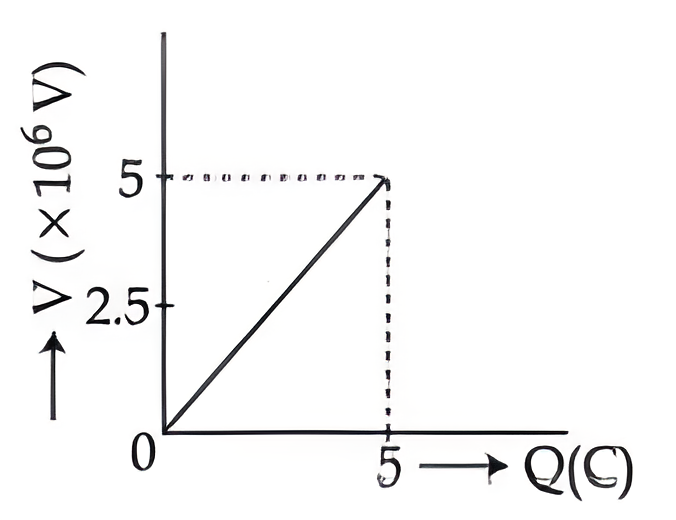
A

B

C

D

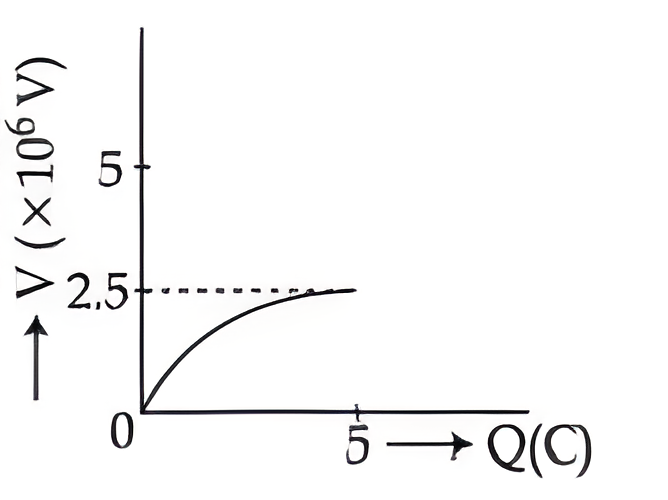
Solution

$Q = CV$
$V =\frac{1}{ C } Q$
Straight line with slope $=\frac{1}{ C }$
Slope $=\frac{1}{C}=\frac{1}{2 \times 10^{-6}}=5 \times 10^5$

A

$8: 5$

B

$5: 4$

C

$5: 3$

D

$8: 7$

Solution

Radius of circular path $R =\frac{\sqrt{2 mk }}{ qB }$
$q =\frac{\sqrt{2 mk }}{ RB }$
$\frac{ q _1}{ q _2}=\sqrt{\frac{ m _1}{ m _2}} \times \frac{ R _2}{ R _1}=\sqrt{\frac{9}{4}} \times \frac{5}{6}=\frac{5}{4}$

A

Source frequency should be increased

B

Another resistance should be added in series with the first resistance.

C

Another capacitor should be added in series with the first capacitor

D

The source frequency should be decreased

Solution

$f=\frac{1}{2 \pi \sqrt{L C}}$
To increase the resonating frequency product of $L$ and $C$ should decrease.
By joining capacitor in series, capacitor will decrease

A

$\frac{2 \sqrt{2} \mu_0 L ^2}{\pi \ell}$

B

$\frac{\mu_0 \ell^2}{2 \sqrt{2} \pi L }$

C

$\frac{2 \sqrt{2} \mu_0 \ell^2}{\pi L }$

D

$\frac{\mu_0 L^2}{2 \sqrt{2} \pi \ell}$

Solution

Assuming current I in outer loop magnetic field at centre $=4 \times \frac{\mu_0 i }{4 \pi \times \frac{ L }{2}} \times\left(2 \sin 45^{\circ}\right)=\frac{2 \sqrt{2} \mu_0 i }{\pi L }$

$M=\frac{\text { Flux through inner loop }}{ i }$
$M =\frac{2 \sqrt{2} \mu_0 \ell^2}{\pi L }$

A

$5 \, pF$

B

$50 \, pF$

C

$100 \, pF$

D

$200\, pF$

Solution

Current in capacitor $I =\frac{ V }{ X _{ C }}$
$I =( V ) \times(\omega C )$
$C =\frac{ I }{ V \omega}=\frac{6.9 \times 10^{-6}}{230 \times 600}=50 \,pF$

A

In primary rainbow, observer sees red colour on the top and violet on the bottom

B

In primary rainbow, observer sees violet colour on the top and red on the bottom

C

In primary rainbow, light wave suffers total internal reflection twice before coming out of water drops

D

Primary rainbow is less bright than secondary rainbow.

Solution

In primary rainbow, red colour is at top and violet is at bottom.
Intensity of secondary rainbow is less in comparison to primary rainbow.

A

$5 \times 10^{-10} v _{ a } m$

B

$5 \times 10^{-10} m$

C

$1.5 \times 10^{-10} m$

D

$5 \times 10^{-10} v _{ B } m$

Solution

$\frac{\mu_{ A }}{\mu_{ B }}=\frac{ c / V _{ A }}{ c / V _{ B }}=\frac{ V _{ B }}{ V _{ A }}=\frac{1}{2}$
Let the thickness is $d$
$\frac{ d }{ v _{ B }}-\frac{ d }{ v _{ A }}=5 \times 10^{-10}$
$d =\frac{5 \times 10^{-10} \times v _{ A } v _{ B }}{ v _{ A }- v _{ B }}$
As $v _{ A }=2 v _{ B } \Rightarrow d =5 \times 10^{-10} \times 2 v _{ B }$
Or $d =5 \times 10^{-10} \times v _{ A }$

A

$1.537 \,eV$

B

$2.46 \,eV$

C

$0.615\, eV$

D

$1.23 \,eV$

Solution

$k _1=\frac{1230}{800}-\phi.....$(1)
$k _2=2 k _1=\frac{1230}{500}-\phi.....$(2)
Eliminating $k _1$ from (1) and (2) we get
$0=\frac{1230}{500}-\frac{1230}{400}+\phi$
$\phi=0.615 \,eV$

A

$\frac{ nh }{2 \pi r }$

B

$\frac{ nh }{2 r }$

C

$\frac{n h}{2 \pi}$

D

$\frac{2 \pi r }{ nh }$

Solution

Angular momentum is integral multiple of $\frac{ h }{2 \pi} mvr =\frac{ nh }{2 \pi}$
So momentum mv $=\frac{ nh }{2 \pi r }$

A

$\vec{\mu}_{ L }=\frac{ e \overrightarrow{ L }}{2 m }$

B

$\vec{\mu}_{ L }=-\frac{e \overrightarrow{ L }}{2 m }$

C

$\vec{\mu}_1=-\frac{e \vec{L}}{m}$

D

$\vec{\mu}_1=\frac{2 e \vec{L}}{m}$

Solution

Ratio of magnetic moment and angular momentum
$\frac{\vec{\mu}}{\overrightarrow{ L }}=\frac{ q }{2 m }$
For $^{-}$
$\vec{\mu}=-\frac{ e }{2 m } \overrightarrow{ L }$

A

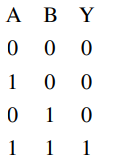
B

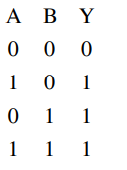
C

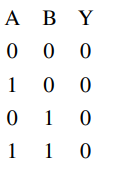
D

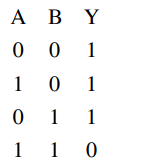
Solution

When both $A$ and $B$ have logical value ' $1$ '
both diode are reverse bias and current will flow in resistor hence output will be $5$ volt i.e. logical value ' $1$ '.
In all other case conduction will take place, hence output will be zero volt i.e. logical value ' $0$ '.
So truth table is

Solution

Stopping distance $=\frac{ v ^2}{2 a }= d$
If speed is made $\frac{1}{3} rd$
$d ^{\prime}=\frac{1}{9} d . \,\,d ^{\prime}=\frac{27}{9}=3$
Braking acceleration remains same

Solution

$\theta=45^{\circ}$
Taking components along $x$ & $y$
$F _1=\sqrt{2}-\frac{1}{\sqrt{2}}=\frac{2-1}{\sqrt{2}}=\frac{1}{\sqrt{2}}$
$F _2=\sqrt{2}+\frac{1}{\sqrt{2}}=\frac{2+1}{\sqrt{2}}=\frac{3}{\sqrt{2}}$
$F _1: F _2=1: 3$
$x =3$

Solution

$\Delta \ell_1=\frac{ F \ell}{ AY }=\frac{ F \ell}{\pi r ^2 Y }=5\, cm$
$\Delta \ell_2=\frac{4 F 4 \ell}{\pi 16 r ^2 Y }=\frac{ F \ell}{\pi r ^2 Y }=5 \,cm$

Solution

$\ell_{ B }\left(1+\alpha_{ B } \Delta T \right)-\ell_{ i }\left(1+\alpha_{ i } \Delta T \right)=\ell_{ B }-\ell_{ i }$
$\alpha_{ B } \ell_{ B }=\ell_{ i } \alpha_{ i }$
$1.8 \times 10^{-5} \times 40=\ell_{ i } \times 1.2 \times 10^{-5}$
$\ell_{ i }=\frac{1.8 \times 10^{-5} \times 40}{1.2 \times 10^{-5}}=\frac{3 \times 40}{2}=60$
$\ell_{ i }=60\, cm$

Solution

$V_S=0, V_{\text {ob }}=5\, m / s$
$f _{\text {direct }}=\left(\frac{320-5}{320}\right) 640=630\, Hz$
$f _{\text {reflected }}=\left(\frac{320+5}{320}\right) 640=650\, Hz$
$f _{\text {beat }}=650-630=20\, Hz$

Solution

No. of electric field lines per unit area $=$ electric field.
$E =\frac{\rho r }{3 \in_0}$, for $r = R$
$E =\frac{\rho R }{3 \epsilon_0}=\frac{2 \times 6}{3 \times 8.85 \times 10^{-12}}=0.45 \times 10^{12} NC ^{-1}$
$=45 \times 10^{10} N / C$

Solution

By nodal analysis
$\frac{ V _0-2}{1 k \Omega}+\frac{ V _0-4}{1 k \Omega}+\frac{ V _0-6}{1 k \Omega}=0$
$3 V _0-12=0$
$V _0=4$

Solution

Each wire has resistance $=\rho \frac{4 \ell}{\pi d ^2}= r$
Eight wire in parallel, then equivalent resistance is $\frac{ r }{8}=\frac{\rho \ell}{2 \pi d ^2}$
Single copper wire of length $2 l$ has resistance
$R =\rho \frac{2 \ell \times 4}{\pi d _1^2}=\frac{\rho \ell}{2 \pi d ^2}$
$\Rightarrow d _1=4 d$

Solution

$E _{ g }=\frac{ hc }{\lambda}=\frac{1242}{\lambda( nm )}=\frac{1242}{400}=3.105$
Answer rounded to $3\, eV$

Solution

$d =\sqrt{2 Rh }$
$d =\sqrt{2 \times 6400 \times h \times 10^{-3}}( h$ in $m )$
Area $=\pi d ^2$
$=\left(\pi \times 2 \times 6400 \times h \times 10^{-3}\right) km ^2$
$6.03 \times 100000=100 \times \pi \times 2 \times 6400 \times 10^{-3} h$
$h =\frac{6.03 \times 10^5}{10 \times \pi \times 128}$
$h =150 \,m$

A

16

B

8

C

4

D

2

Solution

Let $n \left( SO _2 Cl _2\right)= x$ moles
$\therefore n \left( H _2 SO _4\right)= x , n ( HCl )=2 x$
$\Rightarrow n \left( H ^{+}\right)=4 x$
For Neutralisation
$\Rightarrow n \left( H ^{+}\right)= n \left( OH ^{-}\right)$
$\Rightarrow 4 x =16$
$\Rightarrow x =4$

A

$n =3,1=2, m _{ l }=0, s =+\frac{1}{2}$

B

$n =3,1=2, m _1=-2, s =+\frac{1}{2}$

C

$n =3,1=3, m _{ l }=-3, s =-\frac{1}{2}$

D

$n =3,1=0, m _1=0, s =-\frac{1}{2}$

Solution

$1=0,1,2 \ldots \ldots(n-1)$
$\therefore \text { for } n =3$
$1=0,1,2$
$\Rightarrow 1=3$
not possible for $n =3$

A

$0.8$

B

$1.1$

C

$1.9$

D

$2.4$

Solution

${[ HCOOH ]=0.5 \,ml ^{-1}}$
$\Rightarrow\left(0.5\, ml \times 1.05 \,g \,ml ^{-1}\right) HCOOH \text { in } 1 L$
$\Rightarrow 0.525 \,g \,HCOOH$ in $1 L$
$m =\frac{(0.525 / 46)}{1 \,kg } mol$
[Assuming dilute solution]
$\therefore \Delta T _{ f }= iK _{ f } m \Rightarrow i =\frac{\Delta T _{ f }}{ k _{ f } m }=\frac{0.0405 \times 46}{1.86 \times 0.525}=1.9$

A

$3.2$

B

$4.2$

C

$5.2$

D

$6.2$

Solution

$pH =\frac{ pK _{ w }- pK _{ b }-\log C }{2}$
$=\frac{14-5+1.48}{2}=5.24$

A

(A) - (II), (B) - (IV), (C) - (I), (D) - (III)

B

(A) - (II), (B) - (I), (C) - (IV), (D) - (III)

C

(A) - (III), (B) - (IV), (C) - (I), (D) - (II)

D

(A) - (III), (B) - (I), (C) - (IV), (D) - (II)

Solution

Factual

A

Unnilbium

B

Unnilunium

C

Unnilquadium

D

Unniltrium

Solution

Atomic Number 103

A

(3) (4) Only

B

(1), (2), (5) Only

C

(1), (2) Only

D

(2) Only

Solution

$FeO + SiO _2 \rightarrow FeSiO _3$

A

$Mn ^{2+}$

B

$Mn ^{4+}$

C

$Mn ^{3+}$

D

$Mn ^{6+}$

Solution

$H _2 O _2+ MnO _4^{-} \rightarrow Mn ^{2+}+ O _2$ (unbalanced $)$

A

$Li < K < Na < Rb < Cs$

B

$Li < Na < K < Rb < Cs$

C

$Cs < Rb < K < Na < Li$

D

$Li < Na < K < Cs < Rb$

Solution

Factual

A

trigonal planar

B

tetrahedral

C

pyramidal

D

square planar

Solution

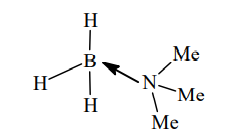
$BF_3 + NaH \xrightarrow{450\,K} \underset{\text{(diborane)}}{B_2H_6} + NaF$
$B_2H_6 + NMe_3 \rightarrow \underset{\text{symmetrical cleavage}}{2[BH_3 \leftarrow NMe_3]}$

A

hypohalite

B

halate

C

perhalate

D

halite

Solution

$Br_2 + \underset{\text{(excess)}}{ 5F_2} \rightarrow \overset{+5}{2BrF_5} \xrightarrow{H_2O} HBrO_3$ (Forms bromate)

A

$NO$

B

$NO _2$

C

$SO _2$

D

$HCHO$

Solution

Factual

A

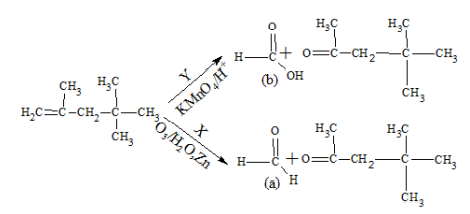
$KMnO _4 / H ^{+}$and dil. $KMnO _4, 273 \,K$

B

$KMnO _4$, (dilute), $273 K$ and $KMnO _4 / H ^{+}$

C

$KMnO _4 / H ^{+}$and $O _3, H _2 O / Zn$

D

$O _3, H _2 O / Zn$ and $KMnO _4 / H ^{+}$

A

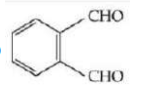
B

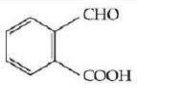
C

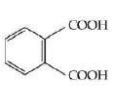
D

A

B

C

D

A

B

C

D

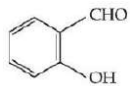
Solution

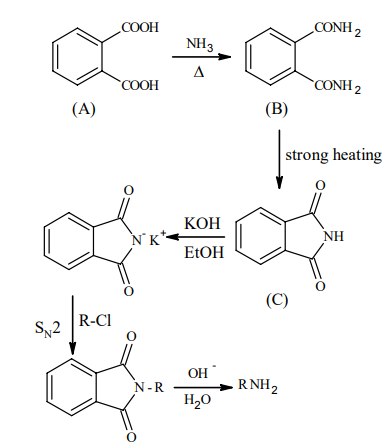
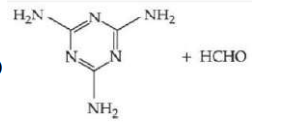
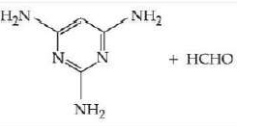
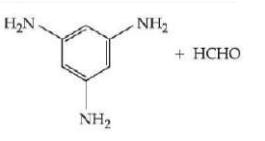
Gabriel Pthalimide reaction

A

B

C

D

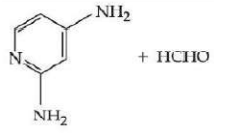
Solution

Formaldehyde $HCHO$
Melamine formaldehyde Resin is melamine polymer

A

Primary

B

Secondary

C

Tertiary

D

Quaternary

Solution

Primary structure remains intact during denaturation of proteins

A

Agonists

B

Antagonists

C

Allosterists

D

Anti histaminists

Solution

Factual

A

Both Statement I and Statement II are correct.

B

Both Statement I and Statement II are incorrect

C

Statement I is correct but Statement II is incorrect.

D

Statement I is incorrect but Statement II is correct.

Solution

Acrolein has a pungent, suffocating odour. Acrolein is used to detect presence of glycerol

Solution

Diamagnetic species are: $N _2, O _2^{2-}$

Solution

$3 C _{( gr )}+4 H _{2( g )} \rightarrow C _3 H _{8( g )}$
$=-103.7 \,kJ\, mol ^{-1}$

Solution

${\left[ P _{ gas }\right]_0+\text { V.P. }=4}$
${\left[ P _{ gas }\right]_0=4-0.4=3.6}$
As volume is doubled, $\left[ P _{ gas }\right]_{\text {new }}=1.8\, atm$
New Total Pressure $=1.8+0.4=2.2 a\,tm$

Solution

$E = E ^0-\frac{2.303 RT }{ nF } \log Q$
Here, $E =+0.801 \,V , E ^0=0.008-(-0.763)$
$=+0.771\, V$
$\therefore 0.801=+0.771-\frac{0.06}{ n } \log 10^{-2}$
$\Rightarrow n =4$

Solution

$\left( t _{1 / 2}\right)_{500 \text { torr }}=240 \,\sec =4\,\min$.
$\left( t _{1 / 2}\right)_{250 \text { torr }}=4 \,\min$.
$t _{1 / 2} \propto a ^{1- n }$
As $t_{1 / 2}$ is independent of initial pressure. Hence, order is $1 st$ order.

Solution

$\Delta_0 \propto \frac{1}{\lambda}$
Here, $CN ^{-}$being SFL will have maximum CFSE
So, $\left[ Co ( CN )_6\right]^{3-}$ will be $d ^2 sp ^3, \mu=0$

Solution

$Co ^{3+}$ can't liberate $H _2$.
It has $d ^6$ configuration,
Number of unpaired electrons $=4$
$\mu=\sqrt{4 \times 6}=4.92 \text { B.M. }$

Solution

$\% N =\frac{1.4\left( N _1 V _1\right)}{\text { massof organic compound }}$
$\% N =\frac{1.4(2.5 \times 2 \times 2)}{0.25}=56$

A

60

B

90

C

108

D

126

Solution

$A =\{1,2,3,4\}$
$B =\{1,2,3,4,5,6\}$
Here $f(3)$ can be $2,3,4,5,6$
$f (3)=2,( f (1), f (2)) \rightarrow(1,1) \rightarrow 6$ cases
$f (3)=3,( f (1), f (2)) \rightarrow(1,2),(2,1)$
$\rightarrow 2 \times 6=12$ cases
$f (3)=4,( f (1), f (2)) \rightarrow(1,3),(3,1),(2,2)$
$\rightarrow 3 \times 6=18$ cases
$f (3)=5,( f (1), f (2)) \rightarrow(1,4),(4,1),(2,3)$
$(3,2)$
$\rightarrow 4 \times 6=24$ cases
$f (3)=6, ( f (1), f (2))$
$(1,5),(5,1),(2,4),(4,2),(3,3)$
$\rightarrow 5 \times 6=30$ cases
Total number of cases $=6+12+18+24+ 30=90$

A

$-4$

B

$-1$

C

1

D

4

Solution

$\alpha, \beta, \gamma, \delta$ root of the equation
$x^4+x^3+x^2+x+1=0$
Which are $5^{\text {th }}$ roots of unity except 1 . then $\alpha^{2021}+\beta^{2021}+\gamma^{2021}+\delta^{2021}=$ $\alpha+\beta+\gamma+\delta=-1$

A

0

B

2

C

3

D

4

Solution

$S_n:|z-(3-2 i)|=\frac{n}{4}$ is a circle center $C _1(3,-2)$ and radius $n / 4$
$T _{ n }:| z -(2-3 i )|=\frac{1}{ n }$ is a circle center $C _2$ $(2,-3)$
and radius $1 / n$
Here $S_n \cap T_n=\phi$
Both circles do not intersect each other
Case-1 : $C _1 C _2> n / 4+1 / n$
$\sqrt{2}>\frac{ n }{4}+\frac{1}{ n }$
then $n =1,2,3,4$
Case-2 : $C _1 C _2<\left|\frac{ n }{4}-\frac{1}{ n }\right|$
$\Rightarrow \sqrt{2} < \left|\frac{n^2-4}{4 n}\right|$
$\Rightarrow n$ has infinite solutions for $n \in N$

A

6

B

7

C

8

D

9

Solution

The system of equation has no solution.
$D = \begin{vmatrix}3 \sin 3 \theta & -1 & 1 \\3 \cos 2 \theta & 4 & 3 \\6 & 7 & 7\end{vmatrix}=0$
$21 \sin 3 \theta+42 \cos 2 \theta-42=0$
$\sin 3 \theta+2 \cos 2 \theta-2=0$
Number of solution is 7 in $(0,4 \pi)$

A

4

B

$-8$

C

$-4$

D

8

Solution

$\displaystyle\lim _{n \rightarrow \infty} n\left(1-\frac{n+1}{n^2}\right)^{\frac{1}{2}}+\alpha n+\beta=0$
$\displaystyle\lim _{n \rightarrow \infty}\left\{11-\frac{1}{2}\left(\frac{n+1}{n^2}\right)+\frac{\left(\frac{1}{2}\right)\left(-\frac{1}{2}\right)}{2 !}\left(\frac{n+1}{n^2}\right)^2+\ldots .\right\}+\alpha n+\beta=0$
$\displaystyle\lim _{n \rightarrow \infty} n-\frac{1}{2}+\frac{1}{n}+\ldots .+n \alpha+\beta=0$
$\alpha=-1, \beta=\frac{1}{2}$
$8(\alpha+\beta)=-4$

A

$\alpha=0$

B

$\alpha=-3$

C

$\alpha \in(-1,0)$

D

$\alpha \in(-3,-1)$

Solution

$f ^{\prime}( x )= e ^{\left(4 x ^3-12 x ^2-180 x +31\right)}\left(12\left( x ^2-2 x +7\right)( x +3)( x -5)+2( x -1)\right)$
$\text { for } x \in[-3,0]$
$\Rightarrow f ^{\prime}( x ) < 0$
$f(x)$ is decreasing function on $[-3, 0]$
The absolute maximum value of the function $f(x)$ is at $x=-3$
$\Rightarrow \alpha=-3$

A

$\frac{27}{4}$

B

$\frac{29}{4}$

C

$\frac{37}{4}$

D

$\frac{9}{2}$

Solution

$y(x)=a x^3+b x^2+c x+5$ is passing through $(-2,0)$ then $8 a-4 b+2 c=5 \ldots \ldots$ (1)
$y^{\prime}(x)=3 a x^2+2 b x+c$ touches $x$-axis at $(- 2,0)$
$12 a-4 b+c=0 .....$(2)
again, for $x=0, y^{\prime}(x)=3$
$c =3 .....$(3)
Solving eq. (1), (2) \& (3) $a =-\frac{1}{2}, b =-\frac{3}{4}$
$y^{\prime}(x)=-\frac{3}{2} x^2-\frac{3}{2} x+3$
$y(x)$ has local maxima at $x=1$
$y(1)=\frac{27}{4}$

A

$\frac{31}{8}$

B

$\frac{17}{6}$

C

$\frac{19}{6}$

D

$\frac{27}{8}$

Solution

$A=\int\limits_{-1}^{\frac{1}{2}}\left(x+2-x^2\right) d x+\int\limits_{\frac{1}{2}}^1\left(4-3 x-x^2\right) d x=\frac{17}{6}$

A

4

B

2

C

1

D

0

Solution

$f(x)$ is periodic function whose period is 2
$\frac{\pi^2}{10} \int\limits_{-10}^{10} f(x) \cos \pi x d x=\frac{\bar{\pi}^2}{10} \times 10 \int\limits_0^2 f(x) \cos \pi x d x$
$=\pi^2\left(\int\limits_0^1(1-x) \cos \pi x d x+\int\limits_1^2(x-1) \cos \pi x d x\right)$
Using by parts
$=\pi^2 \times \frac{4}{\pi^2}=4$

A

$\frac{3+\sqrt{2}}{3-\sqrt{2}}$

B

$\frac{3}{\sqrt{2}}\left(\frac{3+\sqrt{2}}{3-\sqrt{2}}\right)$

C

$\frac{1}{\sqrt{2}}\left(\frac{\sqrt{2}+1}{\sqrt{2}-1}\right)$

D

$\frac{\sqrt{2}+1}{\sqrt{2}-1}$

Solution

$\frac{d y}{d x}=\frac{2 e^{2 x}-6 e^{-x}+9}{2+9 e^{-2 x}}$
$\frac{d y}{d x}=e^{2 x}-\frac{6 e^x}{2 e^{2 x}+9}$
$y=\frac{e^{2 x}}{2}-\tan ^{-1}\left(\frac{\sqrt{2} e^x}{3}\right)+c$
If $C$ passes through the point $\left(0, \frac{1}{2}+\frac{\pi}{2 \sqrt{2}}\right)$
$c=-\frac{\pi}{4}-\tan ^{-1} \frac{\sqrt{2}}{3}$
Again C passes through the point $\left(\alpha, \frac{1}{2} e ^{2 \alpha}\right)$
then $e ^\alpha=\frac{3}{\sqrt{2}}\left(\frac{3+\sqrt{2}}{3-\sqrt{2}}\right)$

A

$\left(y^2+x\right)^4=C\left|\left(y^2+2 x\right)^3\right|$

B

$\left( y ^2+2 x \right)^4= C \left|\left( y ^2+ x \right)^3\right|$

C

$\left|\left(y^2+x\right)^3\right|=C\left(2 y^2+x\right)^4$

D

$\left|\left(y^2+2 x\right)^3\right|=C\left(2 y^2+x\right)^4$

Solution

$\left(x-y^2\right) d x+y\left(5 x+y^2\right) d y=0$
$\frac{d y}{d x}=\frac{y^2-x}{y\left(5 x+y^2\right)} . \text { Let } y^2=v$
$\frac{2 y d y}{d x}=2\left(\frac{y^2-x}{5 x+y^2}\right)$
$\frac{d v}{d x}=2\left(\frac{v-x}{5 x+v}\right) v=k x$
$k + x \frac{ dk }{ dx }=2\left(\frac{ kx - x }{5 x + kx }\right)$
$x \frac{ dk }{ dx }=-\frac{\left( k ^2+3 k +2\right)}{ k +5}$
$\int \frac{(5+k)}{(k+1)(k+2)} d k=\int-\frac{d x}{x}$
$\int\left(\frac{4}{k+1}-\frac{3}{k+2}\right) d k=-\int \frac{d x}{x}$
$4 \ln (k+1)-3 \ln (k+2)=-\ln x+\ln c$
$\frac{(k+1)^4}{(k+2)^3}=-\ln x+\ln c$
$c\left(y^2+2 x\right)^3=\left(y^2+x\right)^4$

A

$2 x+y=9$

B

$3 x-2 y=7$

C

$x+2 y=6$

D

$2 x -3 y =3$

Solution

Let $B\left(x_1, x_1-2\right)$
$\sqrt{\left(x_1-4\right)^2+\left(x_1-2-3\right)^2}=\frac{\sqrt{29}}{3}$
Squaring on both side $18 x _1^2-162 x _1+340=0$
$x _1=\frac{51}{9}$ or $x _1=\frac{10}{3}$
$y _1=\frac{33}{9}$ or $y _1=\frac{4}{3}$
Option (C) will satisfy $\left(\frac{10}{3}, \frac{4}{3}\right)$

A

$\frac{32 \sqrt{2}}{3}$

B

$\frac{40 \sqrt{2}}{3}$

C

$\frac{64}{3}$

D

$\frac{32}{3}$

Solution

$(\alpha-0)^2+(\beta-1)^2=(\beta+1)^2$
$\alpha^2=4 \beta$
$x^2=4 y$
$A=2 \int\limits_0^4\left(4-\frac{x^2}{4}\right) d x=\frac{64}{3}$

A

$\frac{147}{2}$

B

96

C

$\frac{32}{3}$

D

54

Solution

$a ( x -3)+ b ( y +4)+ c ( z -7)=0$
$P : 9 a - b -5 c =0$
$-11 a - b +5 c =0$
After solving DR's $\propto(1,-1,2)$
Equation of plane
$x - y +2 z =21$
$d =\frac{8}{\sqrt{6}}$
$d ^2=\frac{32}{3}$

A

both (S1) and (S2) are true

B

only (S1) is true

C

only (S2) is true

D

both (S1) and (S2) are false

Solution

$\overrightarrow{ a }+\overrightarrow{ b }+\overrightarrow{ c }=0$
$\overrightarrow{ b }+\overrightarrow{ c }=-\overrightarrow{ a }$
$|\overrightarrow{ b }|^2+|\overrightarrow{ c }|^2+2 \overrightarrow{ b } \cdot \overrightarrow{ c }=|\overrightarrow{ a }|^2$
$|\overrightarrow{ c }|^2=36$
$|\overrightarrow{ c }|^2=6$
$S1: |\vec{a} \times \vec{b}+\overrightarrow{ c } \times \overrightarrow{ b }|-|\overrightarrow{ c }|$
$|(\overrightarrow{ a }+\overrightarrow{ c }) \times \overrightarrow{ b }|-|\overrightarrow{ c }|$
$|-\overrightarrow{ b } \times \overrightarrow{ b }|-|\overrightarrow{ c }|$
$0-6=-6$
$S 2: \overrightarrow{ a }+\overrightarrow{ b }+\overrightarrow{ c }=0$
$\overrightarrow{ b }+\overrightarrow{ c }=-\overrightarrow{ a }$
$|\overrightarrow{ a }|^2+|\overrightarrow{ b }|^2-2|\overrightarrow{ a }||\overrightarrow{ b }| \cos (\angle ACB )=|\overrightarrow{ c }|^2$
$\cos (\angle ACB )=\sqrt{\frac{2}{3}}$

A

$\frac{33}{2^{32}}$

B

$\frac{33}{2^{29}}$

C

$\frac{33}{2^{28}}$

D

$\frac{33}{2^{27}}$

Solution

$n p+n p q=24 ....$(1)
$n p \cdot n p q=128....$(2)
Solving (1) and (2):
We get $p =\frac{1}{2}, q =\frac{1}{2}, n =32$.
Now,
$P ( X =1)+ P ( X =2)$
$={ }^{32} C _1 pq ^{31}+{ }^{32} C _2 p ^2 q ^{30}$
$=\frac{33}{2^{28}}$

A

$\frac{17}{36}$

B

$\frac{4}{9}$

C

$\frac{1}{2}$

D

$\frac{19}{36}$

Solution

$x ^2+\alpha x +\beta>0, \forall x \in R$
$D =\alpha^2-4 \beta<0$
$\alpha^2<4 \beta$
Total cases$= 6 \times 6=36$
Fav. cases $=\beta=1, \alpha=1$
$\beta=2, \alpha=1,2$
$\beta=3, \alpha=1,2,3$
$\beta=4, \alpha=1,2,3$
$\beta=5, \alpha=1,2,3,4$
$\beta=6, \alpha=1,2,3,4$
Total favourable cases $=17$
$P ( x )=\frac{17}{36}$

A

4

B

6

C

8

D

12

A

$5(2 \sqrt{3}+3) m$

B

$5(\sqrt{3}+3) m$

C

$10(\sqrt{3}+1) m$

D

$10(2 \sqrt{3}+1) m$

Solution

$\frac{15}{ AQ }=\tan 60^{\circ}$
$\frac{15+x}{ AQ }=\tan 75^{\circ}$
$\frac{(1)}{(2)} \Rightarrow x=10 \sqrt{3}$So, $P Q=5(2 \sqrt{3}+3) m$

A

$((\sim p) \vee q) \Rightarrow p$

B

$p \Rightarrow((\sim p ) \vee q )$

C

$((\sim p ) \vee q ) \Rightarrow q$

D

$q \Rightarrow((\sim p) \vee q)$

Solution

$A= \begin{bmatrix} 2 & -1 & -1 \\1 & 0 & -1 \\ 1 & -1 & 0\end{bmatrix} \Rightarrow A^2=A \Rightarrow A^n=A$
$\forall n \in\{1,2, \ldots, 100\}$
Now, $B = A - I =\begin{bmatrix} 1 & -1 & -1 \\1 & -1 & -1 \\1 & -1 & -1\end{bmatrix}$
$B ^2 =- B$
$\Rightarrow B ^3 =- B ^2= B$
$\Rightarrow B ^5= B$
$\Rightarrow B ^{99}= B$
Also, $\omega^{3 k }=1$
So, $n =$ common of $\{1,3,5, \ldots, 99\}$ and $\{3,6,9, \ldots, 99\}=17$

Solution

$\left( t ^2 x ^{\frac{1}{5}}+\frac{(1- x )^{\frac{1}{10}}}{ t }\right)^{15}$
$T _{ r +1}={ }^{15} C _{ r }\left( t ^2 x ^{\frac{1}{5}}\right)^{15- r } \cdot \frac{(1- x )^{\frac{ r }{10}}}{ t ^{ r }}$
For independent of $t$,
$30-2 r - r =0$
$\Rightarrow r =10$
So, Maximum value of ${ }^{15} C _{10} x (1- x )$ will be at
$x =\frac{1}{2}$
i.e. $6006$

Solution

$x^2-8 a x+2 a=0$
$p+r=8 a$
$p r=2 a$
$\frac{1}{p}+\frac{1}{r}=4$
$\frac{2}{q}=4$
$q=\frac{1}{2}$
$p=\frac{1}{5}$
$x^2+12 b x+6 b=0$
$q+s=-12 b$
$q s=6 b$
$\frac{1}{q}+\frac{1}{s}=-2$
$\frac{2}{r}=-2$
$r =-1$
$s=\frac{-1}{4}$
Now, $\frac{1}{ a }-\frac{1}{ b }=\frac{2}{ pr }-\frac{6}{ qs }=38$

Solution

$a _1= b _1=1$
$a _2= a _1+2=3$
$a _3= a _2+2=5$
$a _4= a _2+2=7$
$\Rightarrow a _{ n }=2 n -1$
$b _2= a _1+ b _1=4$
$b _3= a _3+ b _2=9$
$b _4= a _4+ b _3=16$
$b _{ n }= n ^2$
$\displaystyle\sum_{n=1}^{15} a_n b_n$
$\displaystyle\sum_{n=1}^{15}(2 n-1) n^2$
$\displaystyle\sum_{n=1}^{15}\left(2 n^3-n^2\right)$
$=2 \frac{n^2(n+1)^2}{4}-\frac{n(n+1)(2 n+1)}{6}$
Put $n=15$
$=\frac{2 \times 225 \times 16 \times 16}{4}-\frac{15 \times 16 \times 31}{6}=27560$

Solution

LHS
$\displaystyle\lim _{n \rightarrow \infty} \frac{(n+1)^{k-1}}{n^{k+1}}[n k \cdot n+1+2+\ldots+n]$
$= \displaystyle\lim _{n \rightarrow \infty} \frac{(n+1)^{k-1}}{n^{k+1}} \cdot\left[n^2 k+\frac{n(n+1)}{2}\right]$
$= \displaystyle\lim _{n \rightarrow \infty} \frac{( n +1)^{ k -1} \cdot n ^2\left( k +\frac{\left(1+\frac{1}{ n }\right)}{2}\right)}{ n ^{ k +1}}$
$\Rightarrow \displaystyle\lim _{n \rightarrow \infty}\left(1+\frac{1}{n}\right)\left(k+\frac{\left(1+\frac{1}{n}\right)}{2}\right)$
$\Rightarrow\left(k+\frac{1}{2}\right)$
$\text { RHS }$
$\Rightarrow \displaystyle\lim _{ n \rightarrow \infty} \frac{1}{n^{ k +1}}\left(1^{ k }+2^{ k }+\ldots+ n ^{ k }\right)=\frac{1}{ k +1}$
$\text { LHS }=\text { RHS }$
$\Rightarrow k +\frac{1}{2}=33 \cdot \frac{1}{ k +1}$
$\Rightarrow(2 k +1)( k +1)=66$
$\Rightarrow( k -5)(2 k +13)=0$
$\Rightarrow k =5 \text { or }-\frac{13}{2}$

Solution

$2 p + f -1=0 .....$(1)
$2-p f -4 p =0 .....$(2)
$2=p( f +4)$
$p =\frac{2}{ f +4}$
$2 p =1- f$
$\frac{4}{ f +4}=1- f$
$f ^2+3 f =0$
$f =0 \text { or }-3$
Hyperbola $3 x^2-y^2=3, x^2-\frac{y^2}{3}=1$
$y = mx \pm \sqrt{ m ^2-3}$
It passes $(1,0)$
$o = m \pm \sqrt{ m ^2-3}$
$m$ tends $\infty$
It passes $(1,3)$
$3=m \pm \sqrt{m^2-3}$
$(3-m)^2=m^2-3$
$m=2$

Solution

$x^2=\frac{64.5}{75}\left(y-\frac{3}{5}\right)$
equation of tangent at $\left(\frac{8}{5}, \frac{6}{5}\right)$
$x \cdot \frac{8}{5}=\frac{64}{15}\left(\frac{y+\frac{6}{5}}{2}-\frac{3}{5}\right)$
$3 x-4 y=0$
equation of family of circle is
$\left(x-\frac{8}{5}\right)^2+\left(y-\frac{6}{5}\right)^2+\lambda(3 x-4 y)=0$
It touches $y$ axis so $f ^2= c$
$x^2+y^2+x\left(3 \lambda-\frac{16}{5}\right)+y\left(-4 \lambda-\frac{12}{5}\right)+4=0$
$\frac{\left(4 \lambda+\frac{12}{5}\right)^2}{4}=4$
$\lambda=\frac{2}{5} \text { or } \lambda=-\frac{8}{5}$
$\lambda=\frac{2}{5}, r=1$
$\lambda=-\frac{8}{5}, r=4$
$d_1+d_2=10$

Solution

DR's of line of shortest distance
$\begin{vmatrix} \hat{ i } & \hat{ j } & \hat{ k } \\0 & 1 & 1 \\2 & 2 & 1\end{vmatrix}=-\hat{ i }+2 \hat{ j }-2 \hat{ k }$
angle between line and plane is $\cos ^{-1} \sqrt{\frac{2}{27}}=\alpha$
$\cos \alpha=\sqrt{\frac{2}{27}}, \sin \alpha=\frac{5}{3 \sqrt{3}}$
DR's normal to plane $(1,-1,-1)$
$\sin \alpha=\left|\frac{-a-2+2}{\sqrt{4+4+1} \sqrt{a^2+1+1}}\right|=\frac{5}{3 \sqrt{3}}$
$\sqrt{3}| a |=5 \sqrt{a^2+2}$
$3 a^2=25 a^2+50$
No value of (a)