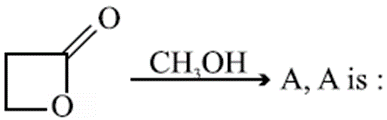
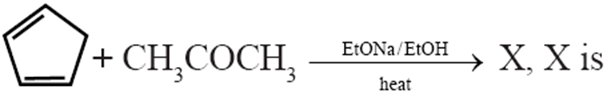Question

6 mol of a mixture of Mohr's salt and $Fe_{2}\left(\right.SO_{4}\left(\left.\right)_{3}$ requires 500 ml of 1 M of $K_{2}Cr_{2}O_{7}$ for complete oxidation in acidic medium. The mole % of the Mohr's salt in the mixture is

• A. 75
• B. 50
• C. 60
• D. 25

Answer 1

Answer: (B)

$Cr_{2}O_{7}^{2 -}+6Fe^{2 +}\left(\right.n=1\left.\right)$ (Mohr's salt) $+14H^{+} \rightarrow 2Cr^{3 +}+6Fe^{3 +}+7H_{2}O$
Equivalent of $Fe^{2 +}=$ moles of Mohr's salt
$=$ equivalent of $K_{2}Cr_{2}O_{7}$
$=500\times 10^{- 3}\times 6\times 1=3$
Hence mole percent of Mohr's salt
$=\frac{3}{6}\times 100=50$