Q. Consider a tap situated on the top of a $5m$ high tower. What is the position of the $2^{nd}$ drop if it is known that, the $3^{rd}$ drop leaves the tap when the $1^{st}$ drop reaches the ground?

NTA AbhyasNTA Abhyas 2020 Report Error

A

$1.25m$

B

$2.50m$

C

$3.75m$

D

$4.00m$

Solution:

$t=\sqrt{\frac{2 h}{g}}=\sqrt{\frac{2 \times 5}{10}}=1s$

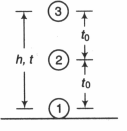
Let t₀ is the interval between two drops. then
2 t₀ = t
t₀ = 0.5 s
2nd drop has taken t0 time to fall. Therefore distance fallen,
$d=\frac{1}{2}gt_{0}^{2}=\left(\frac{1}{2}\right)\left(\right.10\left.\right)\left(\right.0.5\left(\left.\right)^{2}$
= 1.25 m
Height from ground = h - d
= 5 - 1.25
= 3.75 m

Questions from NTA Abhyas 2020

1. A bob of mass $\text{M}$ is suspended by a massless string of length $\text{L}$ . The horizontal velocity $\text{v}$ at position $\text{A}$ is just sufficient to make it reach point $\text{B}$ . The angle $\theta $ at which the speed of the bob is half of that at $\text{A}$ satisfies

Work, Energy and Power

2. The velocity displacement graph of a particle moving along a straight line is -

The most suitable acceleration-displacement graph will be

Motion in a Straight Line

3. A drum of radius $R$ and mass $M$ rolls down without slipping along an inclined plane of angle $\theta $ . The frictional force

System of Particles and Rotational Motion

4. A sample of $^{18} F$ is used internally as a medical diagnostic tool to look for the effects of the positron decay $\left(\right. T_{\frac{1}{2}} = 110 \, \text{min} \left.\right)$ . How long does it take for $\text{99\%}$ of the $^{18}F$ to decay?

Nuclei

5. If the mass of neutron $=1.7\times 10^{- 27}\text{ kg}$ , then the de-Broglie wavelength of neutron of energy $\text{3 eV}$ is $\left(\right.h=6.6\times \left(10\right)^{- 34 \, }J-s\left.\right)$

Physics Most Viewed Questions

1. If $E$ and $G$ respectively denote energy and gravitational constant, then $\frac{ E }{ G }$ has the dimensions of:

NEET 2021 Physical World, Units and Measurements

2. The de Broglie wavelength of an electron moving with kinetic energy of $144 \,eV$ is nearly

NEET 2020 Dual Nature of Radiation and Matter

3. A car starts from rest and accelerates at $5\, m / s ^{2}$ At $t=4\, s$, a ball is dropped out of a window by a person sitting in the car. What is the velocity and acceleration of the ball at $t =6\, s$ ? (Take $\left. g =10\, m / s ^{2}\right)$

NEET 2021 Motion in a Straight Line

4. A wheel with $20$ metallic spokes each $1\, m$ long is rotated with a speed of $120\, rpm$ in a plane perpendicular to a magnetic field of $0.4\, G$. The induced emf between the axle and rim of the wheel will be, $(1 G = 10^{-4}\, T)$

NEET 2020 Electromagnetic Induction

5. A barometer is constructed using a liquid (density $= 760 \,kg/m^{3})$ What would be the height of the liquid column, when a mercury barometer reads $76\, cm$ ?
(density of mercury $= 13600 kg/m^{3})$

NEET 2020 Mechanical Properties of Fluids