- Tardigrade
- Question
- Physics
- A 10.0- kg block is released from rest at point (A) in figure. The track is frictionless except for the portion between points (B) and (C), which has a length of 6.00 m. The block travels down the track, hits a spring of force constant 3000 N / m and compresses the spring 0.20 m from its equilibrium position before coming to rest momentarily. If the coefficient of kinetic friction between the. block and the rough surface between points (B) and (C) is 10 x. Find the value of x.
Q.
A $10.0- kg$ block is released from rest at point (A) in figure. The track is frictionless except for the portion between points (B) and (C), which has a length of $6.00\, m$. The block travels down the track, hits a spring of force constant $3000 \,N / m$ and compresses the spring $0.20\, m$ from its equilibrium position before coming to rest momentarily. If the coefficient of kinetic friction between the. block and the rough surface between points (B) and (C) is $10\, x$. Find the value of $x$.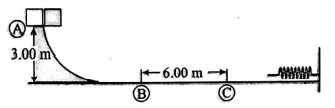
Work, Energy and Power
Report Error
Solution:
The easiest way to solve this problem about a chain-reaction process is by considering the energy changes experienced by the block between the point of release (initial) and the point of full compression of the spring (final). Recall that the change in potential energy (gravitational and elastic) plus the change in kinetic energy must equal the work done on the block by non-conservative forces. We choose the gravitational potential energy to be zero along the flat portion of the track.
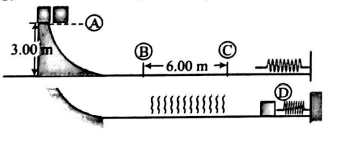
There is zero spring potential energy in situation (A) and (D) ero gravitational potential energy in situation Putting the energy equation into symbols:
$K_D-K_A-U_{g A}+U_{s D}=-f_k d_{B C}$
Expanding into specific variables:
$0-0-m g y_A+\frac{1}{2} k x_s^2=-f_k d_{B C}$
The friction force is $f_k=\mu_k m g d$,
so $m g y_A-\frac{1}{2} k x^2=\mu_k m g d$
Solving for the unknown variable $\mu_k$ gives
$ \mu_k=\frac{y_A}{d}-\frac{k x^2}{2 m g d}$
$ =\frac{3.00 m }{6.00 m }-\frac{(3000 N / m )(0.20 m )^2}{2(10.0 kg )\left(10 m / s ^2\right)(6.00 m )}=0.40$
Hence, $x=4$.
Questions from Work, Energy and Power
Physics Most Viewed Questions
1. If $E$ and $G$ respectively denote energy and gravitational constant, then $\frac{ E }{ G }$ has the dimensions of:
NEET 2021
Physical World, Units and Measurements
2. The de Broglie wavelength of an electron moving with kinetic energy of $144 \,eV$ is nearly
NEET 2020
Dual Nature of Radiation and Matter
3. A car starts from rest and accelerates at $5\, m / s ^{2}$ At $t=4\, s$, a ball is dropped out of a window by a person sitting in the car. What is the velocity and acceleration of the ball at $t =6\, s$ ? (Take $\left. g =10\, m / s ^{2}\right)$
NEET 2021
Motion in a Straight Line
Latest Updates
- JEE Main 2023 February 25th Shift 1 Morning
- JEE Main 2023 February 25th Shift 2 Evening
- JEE Main 2023 January 31st Shift 1 Morning
- JEE Main 2023 January 31st Shift 2 Evening
- JEE Main 2023 January 30th Shift 1 Morning
- JEE Main 2023 January 30th Shift 2 Evening
- JEE Main 2023 January 25th Shift 1 Morning
- JEE Main 2023 January 25th Shift 2 Evening
- JEE Main 2023 January 24th Shift 1 Morning
- JEE Main 2023 January 24th Shift 2 Evening
- JEE Main 2023 February 1st Shift 1 Morning
- JEE Main 2023 February 1st Shift 2 Evening
- JEE Main 2022 July 25th Shift 1 Morning
- JEE Main 2022 July 25th Shift 2 Evening
- JEE Main 2022 July 26th Shift 1 Morning
- JEE Main 2022 July 28th Shift 1 Morning
- JEE Advanced 2022 Paper 2
- JEE Advanced 2022 Paper 1
- JEE Advanced 2021 Paper 2
- JEE Advanced 2021 Paper 1
- JEE Advanced 2020 Paper 2
- JEE Advanced 2020 Paper 1
- NEET 2022 Physics Answer Key
- NEET 2022 Chemistry Answer Key
- NEET 2022 Botany Biology Answer Key
- NEET 2022 Zoology Biology Answer Key
- NEET Rank Predictor 2023