- Tardigrade
- Question
- Physics
- In a cylindrical glass container a solid silica silica is placed vertically at its bottom and remaining space is filled with mercury upto the top level of the silica cylinder as shown in the figure-1.26. Assume that the volume of the silica remains unchanged due to variation in temperature. The coefficient of cubical expansion of mercury is γ and coefficient of linear expansion of glass is α. If the top surface of silica and mercury level remain at the same level with the variation in temperature then the ratio of volume of silica to the volume of mercury is equal to :
Q.
In a cylindrical glass container a solid silica silica is placed vertically at its bottom and remaining space is filled with mercury upto the top level of the silica cylinder as shown in the figure-$1.26$. Assume that the volume of the silica remains unchanged due to variation in temperature. The coefficient of cubical expansion of mercury is $\gamma$ and coefficient of linear expansion of glass is $\alpha$. If the top surface of silica and mercury level remain at the same level with the variation in temperature then the ratio of volume of silica to the volume of mercury is equal to :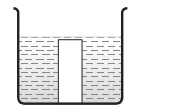
Thermal Properties of Matter
Report Error
Solution:
If $A_{1}$ is cross sectional area of silica cylinder, $\left(A_{1}+A_{2}\right)$ is cross sectional area of glass cylinder, and $h$ is the height of silica cylinder, then :
$h\left(A_{1}+A_{2}\right)(2 \alpha) \Delta \theta=h\left(A_{2}\right) y \Delta \theta$
$\Rightarrow \frac{h A_{1}}{h A_{2}}=\left(\frac{\lambda}{2 \alpha}-1\right)$
$\Rightarrow \frac{\text { volume of silica }}{\text { volume of mercury }}=\left(\frac{\lambda}{2 \alpha}-1\right)$
Questions from Thermal Properties of Matter
1. If the temperature difference on the two sides of a wall increases from $ 100{}^\circ C $ to $ 200{}^\circ C $ , its thermal conductivity
Rajasthan PMT 2007
Physics Most Viewed Questions
1. If $E$ and $G$ respectively denote energy and gravitational constant, then $\frac{ E }{ G }$ has the dimensions of:
NEET 2021
Physical World, Units and Measurements
2. The de Broglie wavelength of an electron moving with kinetic energy of $144 \,eV$ is nearly
NEET 2020
Dual Nature of Radiation and Matter
3. A car starts from rest and accelerates at $5\, m / s ^{2}$ At $t=4\, s$, a ball is dropped out of a window by a person sitting in the car. What is the velocity and acceleration of the ball at $t =6\, s$ ? (Take $\left. g =10\, m / s ^{2}\right)$
NEET 2021
Motion in a Straight Line
Latest Updates
- JEE Main 2023 February 25th Shift 1 Morning
- JEE Main 2023 February 25th Shift 2 Evening
- JEE Main 2023 January 31st Shift 1 Morning
- JEE Main 2023 January 31st Shift 2 Evening
- JEE Main 2023 January 30th Shift 1 Morning
- JEE Main 2023 January 30th Shift 2 Evening
- JEE Main 2023 January 25th Shift 1 Morning
- JEE Main 2023 January 25th Shift 2 Evening
- JEE Main 2023 January 24th Shift 1 Morning
- JEE Main 2023 January 24th Shift 2 Evening
- JEE Main 2023 February 1st Shift 1 Morning
- JEE Main 2023 February 1st Shift 2 Evening
- JEE Main 2022 July 25th Shift 1 Morning
- JEE Main 2022 July 25th Shift 2 Evening
- JEE Main 2022 July 26th Shift 1 Morning
- JEE Main 2022 July 28th Shift 1 Morning
- JEE Advanced 2022 Paper 2
- JEE Advanced 2022 Paper 1
- JEE Advanced 2021 Paper 2
- JEE Advanced 2021 Paper 1
- JEE Advanced 2020 Paper 2
- JEE Advanced 2020 Paper 1
- NEET 2022 Physics Answer Key
- NEET 2022 Chemistry Answer Key
- NEET 2022 Botany Biology Answer Key
- NEET 2022 Zoology Biology Answer Key
- NEET Rank Predictor 2023