Q. Consider a circular loop of radius R on the xy-plane carrying a steady current anticlockwise. The magnetic field at the center of the loop is given by
Solution:
A circular loop of radius $R$ and current $I$ is shown in the figure below

From Biot-savart law, the magnetic field at some point in space at distance R is given as,
$d B=\frac{\mu_{0}}{4 \pi} i \frac{ d l \times P }{R^{3}}$
Since, the loop is circular in shape so,
$=2 \pi R$
Now integrating the field in whole length of wire loop
$\Rightarrow \int_\limits{0}^{B} d B=\frac{\mu_{0}}{4 \pi} \frac{i R}{R^{3}} \int_\limits{0}^{2 \pi R} d l $
$\Rightarrow B=\frac{\mu_{0}}{4 \pi} \frac{i}{R^{2}} 2 \pi R=\frac{\mu_{0} i}{2 R}$
Also, with help of right hand thumb rule, we can conclude, that the magnetic field is in $+ z$ direction.
$\Rightarrow B =\frac{\mu_{0} i}{2 R} \hat{ z }$
Questions from KEAM 2019
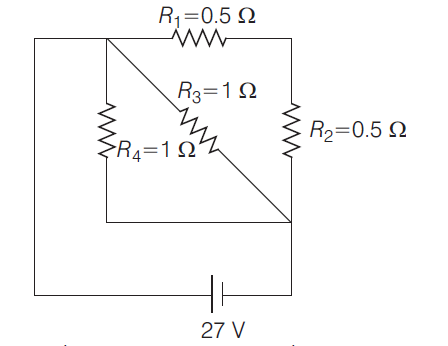
2. A circuit is made using $R_1, R_2, R_3, R_4$ and a battery as shown in the following figure. Find the equivalent resistance of the given circuit and the current passing through $R_3$
Current Electricity
Questions from Moving Charges and Magnetism
Physics Most Viewed Questions
1. If $E$ and $G$ respectively denote energy and gravitational constant, then $\frac{ E }{ G }$ has the dimensions of:
NEET 2021
Physical World, Units and Measurements
2. The de Broglie wavelength of an electron moving with kinetic energy of $144 \,eV$ is nearly
NEET 2020
Dual Nature of Radiation and Matter
3. A car starts from rest and accelerates at $5\, m / s ^{2}$ At $t=4\, s$, a ball is dropped out of a window by a person sitting in the car. What is the velocity and acceleration of the ball at $t =6\, s$ ? (Take $\left. g =10\, m / s ^{2}\right)$
NEET 2021
Motion in a Straight Line
Latest Updates
- JEE Main 2023 February 25th Shift 1 Morning
- JEE Main 2023 February 25th Shift 2 Evening
- JEE Main 2023 January 31st Shift 1 Morning
- JEE Main 2023 January 31st Shift 2 Evening
- JEE Main 2023 January 30th Shift 1 Morning
- JEE Main 2023 January 30th Shift 2 Evening
- JEE Main 2023 January 25th Shift 1 Morning
- JEE Main 2023 January 25th Shift 2 Evening
- JEE Main 2023 January 24th Shift 1 Morning
- JEE Main 2023 January 24th Shift 2 Evening
- JEE Main 2023 February 1st Shift 1 Morning
- JEE Main 2023 February 1st Shift 2 Evening
- JEE Main 2022 July 25th Shift 1 Morning
- JEE Main 2022 July 25th Shift 2 Evening
- JEE Main 2022 July 26th Shift 1 Morning
- JEE Main 2022 July 28th Shift 1 Morning
- JEE Advanced 2022 Paper 2
- JEE Advanced 2022 Paper 1
- JEE Advanced 2021 Paper 2
- JEE Advanced 2021 Paper 1
- JEE Advanced 2020 Paper 2
- JEE Advanced 2020 Paper 1
- NEET 2022 Physics Answer Key
- NEET 2022 Chemistry Answer Key
- NEET 2022 Botany Biology Answer Key
- NEET 2022 Zoology Biology Answer Key
- NEET Rank Predictor 2023
