- Tardigrade
- Question
- Physics
- A thin spherical shell of radius R is cut into two equal halves and then each of its halves is charged to the same uniform surface charge density σ . If F is the force exerted on each half to keep them at equilibrium, then F is proportional to <img class=img-fluid question-image alt=Question src=https://cdn.tardigrade.in/q/nta/p-7aboj6rnalnxen4j.jpg />
Q.
A thin spherical shell of radius $R$ is cut into two equal halves and then each of its halves is charged to the same uniform surface charge density $\sigma $ . If $F$ is the force exerted on each half to keep them at equilibrium, then $F$ is proportional to
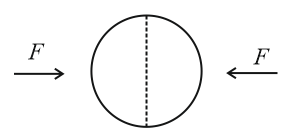
Solution:
Electrical force per unit area $=\frac{1}{2} \varepsilon_{0} E^{2}=\frac{1}{2} \varepsilon_{0}\left(\frac{\sigma}{\varepsilon_{0}}\right)^{2}=\frac{\sigma^{2}}{2 \varepsilon_{0}}$
Projected area $=\pi R^{2}$
$\therefore \quad$ Net electrical force $=\left(\frac{\sigma^{2}}{2 \varepsilon_{0}}\right)\left(\pi R^{2}\right)$
In equilibrium, this force should be equal to the applied force.
$∴$ $\textit{F} = \frac{\pi σ^{2} \textit{R}^{2}}{2 ε_{0}}$
or $\textit{F} ∝ \frac{σ^{2} \textit{R}^{2}}{ε_{0}}$
Questions from NTA Abhyas 2022
5. The force of repulsion between two point charges is $F$ , when these are at distance $0.5 \, m$ apart. Now the point charges are replaced by non conducting spheres of radii $5 \, cm$ each having the same charge as that of the respective point charges. The distance between their centres is again kept $0.5m.$ Then the force of repulsion will
Electrostatic Potential and Capacitance
Physics Most Viewed Questions
1. If $E$ and $G$ respectively denote energy and gravitational constant, then $\frac{ E }{ G }$ has the dimensions of:
NEET 2021
Physical World, Units and Measurements
2. The de Broglie wavelength of an electron moving with kinetic energy of $144 \,eV$ is nearly
NEET 2020
Dual Nature of Radiation and Matter
3. A car starts from rest and accelerates at $5\, m / s ^{2}$ At $t=4\, s$, a ball is dropped out of a window by a person sitting in the car. What is the velocity and acceleration of the ball at $t =6\, s$ ? (Take $\left. g =10\, m / s ^{2}\right)$
NEET 2021
Motion in a Straight Line
Latest Updates
- JEE Main 2023 February 25th Shift 1 Morning
- JEE Main 2023 February 25th Shift 2 Evening
- JEE Main 2023 January 31st Shift 1 Morning
- JEE Main 2023 January 31st Shift 2 Evening
- JEE Main 2023 January 30th Shift 1 Morning
- JEE Main 2023 January 30th Shift 2 Evening
- JEE Main 2023 January 25th Shift 1 Morning
- JEE Main 2023 January 25th Shift 2 Evening
- JEE Main 2023 January 24th Shift 1 Morning
- JEE Main 2023 January 24th Shift 2 Evening
- JEE Main 2023 February 1st Shift 1 Morning
- JEE Main 2023 February 1st Shift 2 Evening
- JEE Main 2022 July 25th Shift 1 Morning
- JEE Main 2022 July 25th Shift 2 Evening
- JEE Main 2022 July 26th Shift 1 Morning
- JEE Main 2022 July 28th Shift 1 Morning
- JEE Advanced 2022 Paper 2
- JEE Advanced 2022 Paper 1
- JEE Advanced 2021 Paper 2
- JEE Advanced 2021 Paper 1
- JEE Advanced 2020 Paper 2
- JEE Advanced 2020 Paper 1
- NEET 2022 Physics Answer Key
- NEET 2022 Chemistry Answer Key
- NEET 2022 Botany Biology Answer Key
- NEET 2022 Zoology Biology Answer Key
- NEET Rank Predictor 2023
