- Tardigrade
- Question
- Physics
- A ring of mass m=1 kg can slide over a smooth vertical rod. A light string attached to the ring passing over a smooth fixed pulley at a distance of L=0.7 m from the rod is shown in the figure. At the other end of the string, mass M=5 kg is attached, lying over a smooth fixed inclined plane of inclination angle 37°. The ring is held in level with the pulley and released. Determine the velocity of ring (in m / s ) when the string makes an angle (α=37°) with the horizontal. [ sin 37°=0.6. ]
Q.
A ring of mass $m=1 \,kg$ can slide over a smooth vertical rod. A light string attached to the ring passing over a smooth fixed pulley at a distance of $L=0.7\, m$ from the rod is shown in the figure. At the other end of the string, mass $M=5\, kg$ is attached, lying over a smooth fixed inclined plane of inclination angle $37^{\circ}$. The ring is held in level with the pulley and released. Determine the velocity of ring (in $m / s$ ) when the string makes an angle $\left(\alpha=37^{\circ}\right)$ with the horizontal. $\left[\sin 37^{\circ}=0.6\right.$ ]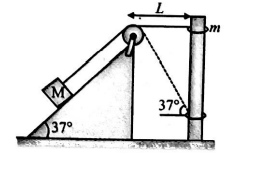
Work, Energy and Power
Report Error
Solution:
Let $x$ is the vertical distance covered by the ring. Then
$x=L \tan 37^{\circ}=0.7 \times \frac{3}{4}$
$\Delta l=L \sec 37^{\circ}-L=L\left(\sec 37^{\circ}-1\right)$
$\Rightarrow \frac{L}{4}=\Delta l$

$\Delta l=$ distance moved by block $M$
Now, from constraint relation
$v_{M}=v_{r} \cos 37^{\circ}=\frac{4}{5} v_{r}$ ...(ii)
$v_{r}=$ velocity of ring,
$v_{M}=$ velocity of the block at this instant
From work-energy theorem, we get $W_{\text {gravity }}=\Delta KE$
$-m g x+M g \Delta l \sin 37^{\circ}+\frac{1}{2} m v_{r}^{2}+\frac{1}{2} M v_{M}^{2}=0$ ...(iii)
On solving equations (i) and (ii), we get
$v{r}=0\, m / s$
Questions from Work, Energy and Power
Physics Most Viewed Questions
1. If $E$ and $G$ respectively denote energy and gravitational constant, then $\frac{ E }{ G }$ has the dimensions of:
NEET 2021
Physical World, Units and Measurements
2. The de Broglie wavelength of an electron moving with kinetic energy of $144 \,eV$ is nearly
NEET 2020
Dual Nature of Radiation and Matter
3. A car starts from rest and accelerates at $5\, m / s ^{2}$ At $t=4\, s$, a ball is dropped out of a window by a person sitting in the car. What is the velocity and acceleration of the ball at $t =6\, s$ ? (Take $\left. g =10\, m / s ^{2}\right)$
NEET 2021
Motion in a Straight Line
Latest Updates
- JEE Main 2023 February 25th Shift 1 Morning
- JEE Main 2023 February 25th Shift 2 Evening
- JEE Main 2023 January 31st Shift 1 Morning
- JEE Main 2023 January 31st Shift 2 Evening
- JEE Main 2023 January 30th Shift 1 Morning
- JEE Main 2023 January 30th Shift 2 Evening
- JEE Main 2023 January 25th Shift 1 Morning
- JEE Main 2023 January 25th Shift 2 Evening
- JEE Main 2023 January 24th Shift 1 Morning
- JEE Main 2023 January 24th Shift 2 Evening
- JEE Main 2023 February 1st Shift 1 Morning
- JEE Main 2023 February 1st Shift 2 Evening
- JEE Main 2022 July 25th Shift 1 Morning
- JEE Main 2022 July 25th Shift 2 Evening
- JEE Main 2022 July 26th Shift 1 Morning
- JEE Main 2022 July 28th Shift 1 Morning
- JEE Advanced 2022 Paper 2
- JEE Advanced 2022 Paper 1
- JEE Advanced 2021 Paper 2
- JEE Advanced 2021 Paper 1
- JEE Advanced 2020 Paper 2
- JEE Advanced 2020 Paper 1
- NEET 2022 Physics Answer Key
- NEET 2022 Chemistry Answer Key
- NEET 2022 Botany Biology Answer Key
- NEET 2022 Zoology Biology Answer Key
- NEET Rank Predictor 2023