- Tardigrade
- Question
- Physics
- A ring of mass M and radius R lies in x-y plane with its centre at origin as shown. The mass distribution of ring is non uniform such that, at any point P on the ring, the mass per unit length is given by λ=λ0 cos 2 θ (where λ0 is a positive constant). Then the moment of inertia of the ring about z -axis is:
Q.
A ring of mass and radius lies in plane with its centre at origin as shown. The mass distribution of ring is non uniform such that, at any point on the ring, the mass per unit length is given by (where is a positive constant). Then the moment of inertia of the ring about -axis is:
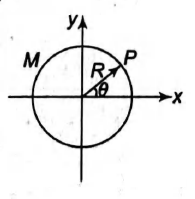
Solution: