A bead slides without friction around a loop-the-loop (Fig.). The bead is released from rest at a height h = 3.50R. How large is the normal force on the bead at point (A) if its mass is 50 g?

Question

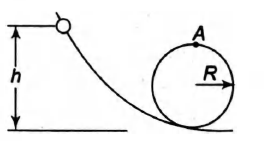
A bead slides without friction around a loop-the-loop (Fig.). The bead is released from rest at a height h = 3.50R. How large is the normal force on the bead at point (A) if its mass is 50 g? <img class="img-fluid question-image" alt="image" src="https://cdn.tardigrade.in/img/question/physics/3b2b189518dea10e8e565665855e5039-.png" /> (A) 0.10 N downward (B) 0.10 N upward (C) 1.0 N downward (D) 1.0 N upward

Tardigrade Admin · Accepted Answer

The speed at the top can be found from the conservation of energy for the bead-track-Earth system,
We define the bottom of the loop as the zero level for the gravitational potential energy.
Since

v_{i} = 0, E_{i} = K_{i} + U_{i} = 0 + m g h = m g (3.50 R)

The total energy of the bead at point (A) can be written as

E_{A} = K_{A} + U_{A} = \frac{1}{2} m v_{A}^{2} + m g (2 R)

Slice mechanical energy is conserved,

E_{i} = E_{A,}

we get

m g (3.50 R) = \frac{1}{2} m v_{A}^{2} + m g (2 R)

simplifying,

v_{A}^{2} = 3.0 g R

\Rightarrow v_{A} = 3.0 g R

To find the normal force at the top, we construct a force diagram as shown,

Here we assume that

N

is downward, like mg.

Σ F_{y} = m a_{y} : N + m g = \frac{m v ^{2}}{r}

N = m [\frac{v ^{2}}{R} - g]

= m [\frac{3.0 g R}{R} - g] = 2.0 m g

N = 2.0 (50 \times 1 0^{- 3} k g) (10.0 m / s^{2})

= 1.0 N

downward

Q. A bead slides without friction around a loop-the-loop (Fig.). The bead is released from rest at a height h=3.50R. How large is the normal force on the bead at point (A) if its mass is 50 g?

0.10 N downward

0.10 N upward

1.0 N downward

1.0 N upward

Q. A bead slides without friction around a loop-the-loop (Fig.). The bead is released from rest at a height $h = 3.50 R$ . How large is the normal force on the bead at point (A) if its mass is 50 g?