Q.
Match the rate expressions in LIST-I for the decomposition of $X$ with the corresponding profiles provided in LIST-II. $X _{ s }$ and $k$ constants having appropriate units.
LIST I
LIST II
I
$\text { rate }=\frac{ k [ X ]}{ X _{ s }+[ X ]}$
under all possible initial concentration of $X$
i
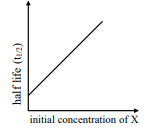
II
$ \text { rate }=\frac{ k [ X ]}{ X _{ s }+[ X ]} $
where initial concentration of $X$ are much less than $X _s$
ii
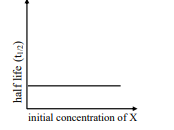
III
$ \text { rate }=\frac{ k [ X ]}{ X _{ s }+[ X ]} $
where initial concentration of $X$ are much higher than $X _{ s }$
iii
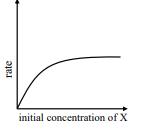
IV
$ \text { rate }=\frac{ k [ X ]^2}{ X _{ s }+[ X ]} $ ,br/>where initial concentration of $X$ is much higher than $X _{ s }$
iv
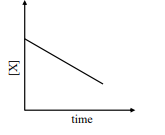
v
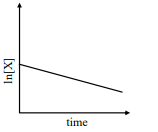
| LIST I | LIST II | ||
|---|---|---|---|
| I | $\text { rate }=\frac{ k [ X ]}{ X _{ s }+[ X ]}$ under all possible initial concentration of $X$ |
i |  |
| II | $ \text { rate }=\frac{ k [ X ]}{ X _{ s }+[ X ]} $ where initial concentration of $X$ are much less than $X _s$ |
ii |  |
| III | $ \text { rate }=\frac{ k [ X ]}{ X _{ s }+[ X ]} $ where initial concentration of $X$ are much higher than $X _{ s }$ |
iii |  |
| IV | $ \text { rate }=\frac{ k [ X ]^2}{ X _{ s }+[ X ]} $ ,br/>where initial concentration of $X$ is much higher than $X _{ s }$ | iv |  |
| v |  |
||
JEE AdvancedJEE Advanced 2022
Solution: