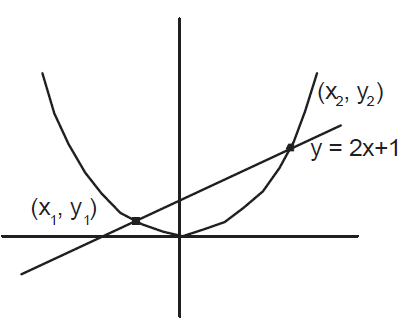Q. If the parabola $x^2 = ay$ makes an intercept of length $\sqrt{40}$ unit on the line $y - 2x = 1$ then a is equal to
WBJEEWBJEE 2016
Solution:
Solving $x^2 = ay$ with $y - 2x = 1$,
$x^{2}=a\left(1+2x\right) \Rightarrow x^{2}-2ax-a=0$
Let $x_1$ & $x_2$ are the roots
so, $\left(x_{1}-x_{2}\right)^{2}=\left(2a\right)^{2}-4\left(-a\right)=4a\left(a+1\right)$
also, $\left(y_{1}-y_{2}\right)^{2}=\left(\left(2x_{1}+1\right)-\left(2x_{2}+1\right)^{2}=4\left(x_{1}-x_{2}\right)^{2}=16a\left(a+1\right)\right)$
now $\sqrt{\left(x_{1}-x_{2}\right)^{2}+\left(y_{1}-y_{2}\right)^{2}}=\sqrt{4a\left(a+1\right)+16a\left(a+1\right)}=\sqrt{40}$
$\Rightarrow 20a\left(a+1\right)=40 \Rightarrow a^{2}+a-2=0 \Rightarrow a^{2}+a-2=0 \Rightarrow a=-2,1$