Q.
Consider a system of two masses and a pulley shown in the figure. The coefficient of friction between the two blocks and also between block and table is $0.1 .$ Find the force $F$, that must be given to the $0.8\, kg$ block such that it attains acceleration of $5\, m / s ^{2}$. (Assume, acceleration due to gravity, $\left.g=10\, m / s ^{2} .\right)$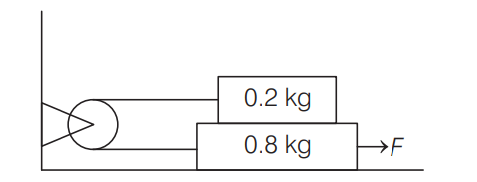
TS EAMCET 2019
Solution:


