Q.
A particle is moving eastwards with a speed of $6\, m / s$. After $6\, s$, the particle is found to be moving with same speed in a direction $60^{\circ}$ north of east. The magnitude of average acceleration in this interval of time is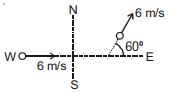
Motion in a Plane
Solution:
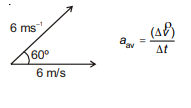
$|\Delta \vec{v}|=2 v \sin \frac{\theta}{2}=2 v \sin \left(\frac{60}{2}\right)=2 v \sin 30^{\circ}=v$
$|\Delta \vec{v}|=6\, ms ^{-1}$
$\Delta t=6\, s$
so, $a_{ av }=\frac{6}{6}=1\, ms ^{-2}$