- Tardigrade
- Question
- Physics
- In a thermodynamic process on an ideal monatomic gas, the infinitesimal heat absorbed by the gas is given by TΔX, where T is temperature of the system and ΔX is the infinitesimal change in a thermodynamic quantity X of the system. For a mole of monatomic ideal gas X = (3/2) R In ((T/TA))+R In ((V/VA)). Here, R is gas constant, V is volume of gas, TA and VA are constants. The List-I below gives some quantities involved in a process and List-II gives some possible values of these quantities. List-I List-II (I) Work done by the system in process 1→2→3 (p) (1/3)RT0 In 2 (II) Change in internal energy in process 1→2→3 (Q) (1/3)RT0 (III) Heat absorbed by the system in process 1→2→3 (R) RT0 (IV) Heat absorbed by the system in process 1→2 (S) (4/3)RT0 (T) (1/3)RT0 (3+In 2) (U) (5/6)RT0 Question: If the process carried out on one mole of monatomic ideal gas is as shown in figure in the PV-diagram with P0 V0 = (1/3)RT0, the correct match is.
Q.
In a thermodynamic process on an ideal monatomic gas, the infinitesimal heat absorbed by the gas is given by TX, where T is temperature of the system and X is the infinitesimal change in a thermodynamic quantity X of the system. For a mole of monatomic ideal gas . Here, R is gas constant, V is volume of gas, and are constants.
The List-I below gives some quantities involved in a process and List-II gives some possible values of these quantities.
List-I
List-II
(I) Work done by the system in process 123
(p)
(II) Change in internal energy in process 123
(Q)
(III) Heat absorbed by the system in process 123
(R)
(IV) Heat absorbed by the system in process 12
(S)
(T)
(U)
Question: If the process carried out on one mole of monatomic ideal gas is as shown in figure in the PV-diagram with the correct match is.
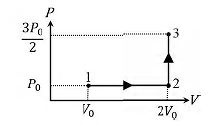
| List-I | List-II |
|---|---|
| (I) Work done by the system in process 123 | (p) |
| (II) Change in internal energy in process 123 | (Q) |
| (III) Heat absorbed by the system in process 123 | (R) |
| (IV) Heat absorbed by the system in process 12 | (S) |
| (T) | |
| (U) |
Solution: