- Tardigrade
- Question
- Physics
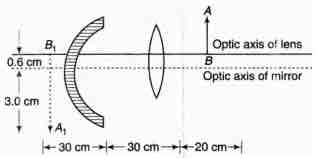
- A convex lens of focal length 15 cm and a concave mirror of focal length 30 cm are kept with their optic axis PQ and RS parallel but separated in vertical direction by 0.6 cm as shown. The distance between the lens and mirror is 30 cm. An upright object AB of height 1.2 cm is placed on the optic axis PQ of the lens at a distance of 20 cm from the lens. If A' B' is the image after refraction from the lens and the reflection from the mirror, find the distance of A'B' from the pole of the mirror and obtain its magnification. Also locate positions of A' and B' with respect to the optic axis RS.
Q.
A convex lens of focal length 15 cm and a concave mirror of
focal length 30 cm are kept with their optic axis PQ and RS
parallel but separated in vertical direction by 0.6 cm as
shown.
The distance between the lens and mirror is 30 cm. An
upright object AB of height 1.2 cm is placed on the optic axis
PQ of the lens at a distance of 20 cm from the lens. If A' B' is
the image after refraction from the lens and the reflection
from the mirror, find the distance of A'B' from the pole of
the mirror and obtain its magnification. Also locate positions
of A' and B' with respect to the optic axis RS.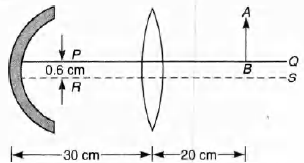
Solution:
(a) Rays coming from object AB first refract from the lens
and then reflect from the mirror.
Refraction from the lens
- 20 cm, f = +15 cm
Using lens formula
v = + 60 cm
and linear magnification,
i.e. first image formed by the lens will be at 60 cm from it
(or 30 cm from mirror) towards left and 3 times
magnified but inverted. Length of first image
would be 1.2 x 3 = 3.6 cm (inverted).
Reflection from mirror Image formed by lens
will behave like a virtual object for mirror at a distance of
30 cm from it as shown. Therefore u = +30 cm,
f = - 30 cm.
Using mirror formula,
v = - 15 cm
and linear magnification,
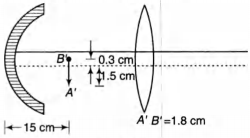
i.e. final image A'B' will be located at a distance of 15 cm
from the mirror (towards right) and since magnification is
, length of final image would be 3.6 x =1.8 cm
A' B' = 1.8 cm
Point is 0.6 cm above the optic axis of mirror,
therefore, its image B' would be (0.6) = 0.3 cm
above optic axis. Similarly, point is 3 cm below the
optic axis, therefore, its image A' will be 3 x = 1.5 cm
below the optic axis as shown below
Total magnification of the image,
A ' B ' = (m )(AB) = -1.8 cm
Note that, there is no need of drawing the ray diagram if
not asked in the question
NOTE With reference to the pole of an optical instrument (whether it
is a lens or a mirror) the coordinates of the object () are
generally known to us. The corresponding coordinates of image
() are found as follows
is obtained using
Here, vis actually and u is ie, the above formula can be written
as
Similarly, is obtained from m=
Here, l is and 0 is i.e., the above formula can be written as