Q.
Uniform thin rod $AB$ of length $L$ and mass $m$ is undergoing fixed axis rotation whose axis of rotation passes through $A$ , such that end $A$ remains stationary as shown. The kinetic energy of section $AP$ of rod is equal to kinetic energy of section $BP$ of the rod at a given instant. Then the ratio of length $AP$ and length $AB$ , that is, $\frac{AP}{AB}$ is equal to
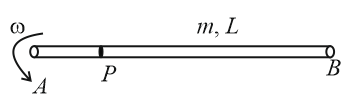
NTA AbhyasNTA Abhyas 2022
Solution:
