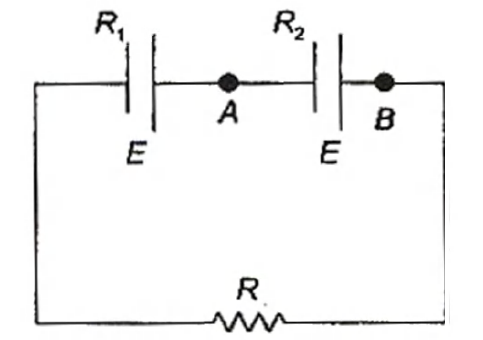
Question

Two sources of equal emf are connected to an external resistance $R$. The internal resistances of the two sources are $R_1$ and $R_2 (R_2 > R_1).$ If the potential difference across the source having internal resistance $R_2$, is zero, then :

• A. $R=\frac{R_{2}\times\left(R_{1}+R_{2}\right)}{\left(R_{2}-R_{1}\right)}$
• B. $R=R_{2}-R_{1}$
• C. $R=\frac{R_{1}R_{2}}{\left(R_{1}+R_{2}\right)}$
• D. $R=\frac{R_{1}R_{2}}{\left(R_{1}-R_{2}\right)}$

Answer 1

Answer: (B)

$R_{eq}=R_{1}+R_{2}+R$
$\therefore I=\frac{2\,E}{R_{1}+R_{2}+R}$
According to the question,
$-\left(V_{A}-V_{B}\right)=E-I\,R_{2}$
$0=E-I\,R_{2}$
$E=I\,R_{2}$
$E=\frac{2\,E}{R_{1}+R_{2}+R}R_{2}$
$R_{1}+R_{2}+R=2R_{2}$
$\therefore r=R_{2}-R_{1}$