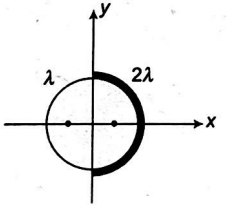
Q. Two semicircular rings of linear mass densities $\lambda$ and $2 \lambda$ and of radius ' $R$ ' each are joined to form a complete ring. The distance of the center of the mass of complete ring from its centre is
System of Particles and Rotational Motion
Solution:
Let the two half rings be placed in left and right of $y$-axis with centre as shown in figure.
Then the coordinate of centre of mass of
left and right half rings are $\left(-\frac{2 R}{\pi}, 0\right)$ and $\left(\frac{2 R}{\pi}, 0\right)$.
$\therefore x$-coordinates of centre of mass of complete ring is
$\frac{m\left(-\frac{2 R}{\pi}\right)+2 m\left(\frac{2 R}{\pi}\right)}{3 m}=\frac{2 R}{3 \pi}$