Question

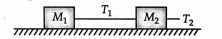
Two masses $M_{1}$ and $M_{2}$ are accelerated uniformly on a frictionless surface as shown in figure. The ratio of the tensions $\frac{T_{1}}{T_{2}}$ is

• A. $\frac{M_{1}}{M_{2}}$
• B. $\frac{M_{2}}{M_{1}}$
• C. $\frac{\left(M_{1}+M_{2}\right)}{M_{2}}$
• D. $\frac{M_{1}}{\left(M_{1}+M_{2}\right)}$

Answer 1

Answer: (D)

Let $a$ be the common acceleration of the system. The equations of motion of masses $M_{1}$ and $M_{2}$ are $T_{1}=M_{1} a \,\,\,\,\, ... (i)$
$T_{2}-T_{1}=M_{2} {a} \,\,\,\,\, ....(ii)$
Adding $(i)$ and $(ii)$, we get $T_{2}=\left(M_{1}+M_{2}\right) a$
$\therefore \frac{T_{1}}{T_{2}}=\frac{M_{1}}{M_{1}+M_{2}}$