Q.
Two masses $ A $ and $ B $ of $ 15\, kg $ and $ 10\, kg $ are connected with a string passing over a frictionless pulley fixed at the corner of a table (as shown in figure). The coefficient of friction between the table and block is $ 0.4 $ . The minimum mass of $ C $ , that may be placed on $ A $ to prevent it from moving is :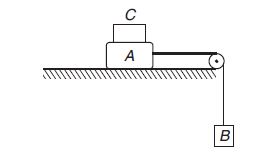
UPSEEUPSEE 2005
Solution:
