Question

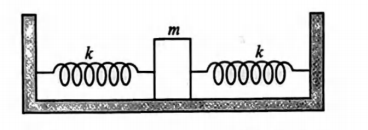
Two identical springs of spring constant $k$ are attached to a block of mass $m$ and to fixed supports as shown in the figure. The time period of oscillation is

• A. $2\pi\sqrt{\frac{m}{k}}$
• B. $2\pi\sqrt{\frac{m}{2k}}$
• C. $2\pi\sqrt{\frac{2m}{k}}$
• D. $\pi\sqrt{\frac{m}{2k}}$

Answer 1

Answer: (B)

Let the mass $m$ be displaced by a small distance $x$ to the right from its mean position as shown in figure $(b)$. Due to it the spring on the left side gets stretched by a length $x$ while that on the right side gets compressed by the same length. The forces acting on the mass are
$F_1 = - k x$ towards left hand side
$F_2 = - k x$ towards left hand side
The net force acting on the mass is, $F = F_1 + F_2 = -2kx$
Here, $F \propto x$ and $-ve$ sign shows that force is towards the mean position, therefore the motion executed by the particle is simple harmonic. Its acceleration is
$a=\frac{F}{m} = -\frac{2kx}{m} \quad...\left(i\right) $
The standard equation of $SHM$ is
$ a=-\omega^{2}x \quad...\left(ii\right)$
Comparing $\left(i\right)$ and $\left(ii\right)$, we get
$\omega^{2} = \frac{2k}{m}$ or $\omega = \sqrt{\frac{2k}{m}} $
Time period, $T= \frac{2\pi}{\omega} = 2\pi\sqrt{\frac{m}{2k}}$