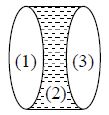
Q. Two identical glass $(\mu_g = 3/2)$ equiconvex lenses of focal length $f$ each are kept in contact. The space between the two lenses is filled with water $(\mu_w = 4/3)$. The focal length of the combination is
Solution:
For equiconvex lens
$\frac{1}{A} = \left(\frac{3}{2} -1 \right) \left(\frac{1}{R} - \frac{1}{-R}\right) $
$ \frac{1}{f } = \frac{1}{2} . \frac{2}{R} $
$ R =f $
For $2^{nd}$ lens $ \frac{1}{f_{2}} = \left(\frac{4}{3} -1\right) \left(\frac{1}{-f} - \frac{1}{-f}\right) $
$ \frac{1}{f_{2} } = \frac{1}{3} \times\frac{2}{-f} $
$ f_{2} = \frac{-3 f}{2} $
For combination
$ \frac{1}{f'} = \frac{1}{f_{1}} + \frac{1}{f_{2}} + \frac{1}{f_{3}} $
$= \frac{1}{f} + \frac{2}{-3 f} + \frac{1}{f} $
$\frac{1}{f'} = \frac{3-2+3}{3f} = \frac{4}{3f}$
$ f' = \frac{3f}{4} $