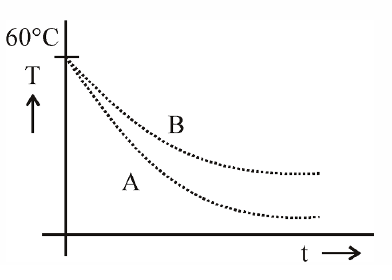
Q. Two identical breakers A and B contain equal volumes of two different liquids at $60^{\circ}C$ each and left to cool down. Liquid in A has density of $8 \times 10^2 \; kg/m^3$ and sp ecific heat o f 2000 J $kg^{-1} \; K^{-1}$ while liquid in B has density o f 103 kg m-3 and sp ecific heat o f 4000 J $kg^{-1} K^{-1}$. Which of the following best describes their temperature versus time graph schematically? (assume the emissivity of both the beakers to be the same)
Solution:
$-ms \frac{dT}{dt} =e\sigma A \left(T^{4} -T_{0}^{4}\right) $
$ -\frac{dT}{dt} = \frac{e\sigma A}{ms} \left(T^{4}-T^{4}_{0}\right) $
$- \frac{dT}{dt} = \frac{4e\sigma AT_{0}^{3}}{ms} \left(\Delta T\right) $
$ T =T_{0} + \left(T_{i} - T_{0}\right)e^{-kt} k = \frac{4e\sigma AT_{0}^{3}}{ms} $
where $ k = \frac{4e\sigma AT_{0}^{3}}{\rho vs} $
$\left|\frac{dT}{dt}\right| \propto k $
$\therefore \left|\frac{dT}{dt}\right|\propto \frac{1}{\rho s} $
$ \rho_{A}S_{A} =2000\times8\times10^{2} =16 \times10^{5} $
$ \rho_{B}S_{B} =4000 \times10^{3} = 4 \times10^{6} $
$ \rho_{A}S_{A} < \rho_{B}S_{B} $
$ \left|\frac{dT}{dt}\right|_{A} > \left|\frac{dT}{dt}\right|_{B} $