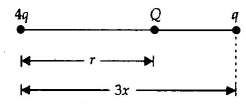
Q. Two charges $4q$ and $q$ are placed $3x$ apart in air $A$ charge $Q$ is placed at a point on the line joining so that the systein of these charges remains in equilibrium. Then, the distance of $Q$ from $4q$ and the value of $Q$ are
Solution:
Given situation is shown in the figure.
To be all charges in equilibrium charge $Q$ should be negative and placed between $q$ and $4q$.
At equilibrium, force between $4q$ and $Q$ = force between $Q$ and $q$.
$\therefore \, \, k \frac{4q .Q}{r^{2}} = k \frac{q .Q}{\left(3x - r\right)^{2}}$
or, $ \frac{4}{r^{2}} = \frac{1}{\left(3x - r\right)^{2}} $
or, $\frac{2}{r} = \frac{1}{3x -r}$
or, $6x - 2r = r $
or, $3r = 6x $
or, $ r = 2x. $
Again, force between $4q$ and $Q$ = force between $q$ and $4q$
$ k \frac{4q.Q}{\left(2x\right)^{2}} = k \frac{4q.q}{\left(3x\right)^{2}} $
or, $ \frac{Q}{4}= \frac{q}{9} or, Q = \frac{4q}{9} $
So charge $ Q = - \frac{4q}{9} r = 2x$