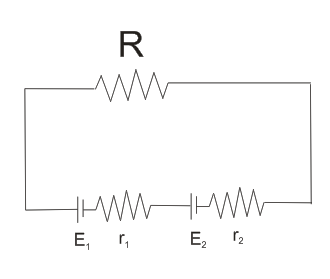
Q. Two cells, having the same E.M.F. are connected in series through an external resistance $R$ . Cells have internal resistances $r_{1}$ and $r_{2}$ $\left(r_{1} > r_{2}\right)$ respectively. When the circuit is closed, the potential difference across the first cell is zero. The value of $R$ is
NTA AbhyasNTA Abhyas 2022
Solution:
Current in the circuit
$=\frac{E + E}{r_{1} + r_{2} + R}=\frac{2 E}{r_{1} + r_{2} + R}$
The potential difference across the first cell $V_{1}=E-ir_{1}$
$\Rightarrow V_{1}=E-\frac{2 E \times r_{1}}{\left(r_{1} + r_{2}\right) + R}$
Now, $E-\frac{2 E r_{1}}{\left(r_{1} + r_{2}\right) + R}=0$
$\Rightarrow E=\frac{2 E r_{1}}{\left(r_{1} + r_{2}\right) + R}\Rightarrow 2r_{1}=r_{1}+r_{2}+R$
$\Rightarrow R=r_{1}-r_{2}$
$=\frac{E + E}{r_{1} + r_{2} + R}=\frac{2 E}{r_{1} + r_{2} + R}$
The potential difference across the first cell $V_{1}=E-ir_{1}$
$\Rightarrow V_{1}=E-\frac{2 E \times r_{1}}{\left(r_{1} + r_{2}\right) + R}$
Now, $E-\frac{2 E r_{1}}{\left(r_{1} + r_{2}\right) + R}=0$
$\Rightarrow E=\frac{2 E r_{1}}{\left(r_{1} + r_{2}\right) + R}\Rightarrow 2r_{1}=r_{1}+r_{2}+R$
$\Rightarrow R=r_{1}-r_{2}$
