Q.
Two balls each of mass $m$ are placed on the vertices $A$ and $B$ of an equilateral $\triangle A B C$ of side $1\, m$. A ball of mass $2\, m$ is placed at vertex $C$. The centre of mass of this system from vertex $A$ (located at origin) is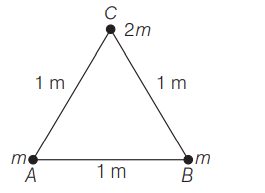
System of Particles and Rotational Motion
Solution:
