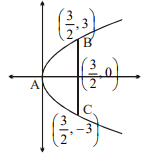
Q. Triangle $ABC$ is inscribed in the parabola described by the equation $y ^2-6 x -4 y +10=0$ so that $A$ is the vertex of the parabola and $B$ and $C$ are the end points of the latus rectum of the parabola. The area of triangle $A B C$ is
Conic Sections
Solution:
$(y-2)^2-4=6\left(x-\frac{5}{3}\right)=6 x-10$
$(y-2)^2=6 x-6=6(x-1)$
Let $y-2=Y$ and $x-1=X$
$\therefore Y ^2=6 X$
Area of $\triangle ABC$,
$=\frac{3}{2} \cdot 6 \cdot \frac{1}{2}=\frac{9}{2} $