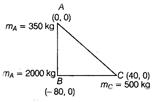
Q. Three uniform spheres, with masses $m_{A}=350\, kg , m_{B}=2000\, kg$, and $m_{C}=500 \,kg$ have the $( x , y )$ coordinates $(0,0) cm ,(-80,0) cm$ and $(40,0) cm$ respectively. The gravitational potential energy, $U$, of the system and change in its value in terms of increase or decrease, if the sphere of mass is removed, may be given as
AMUAMU 2013Gravitation
Solution:
$U=-G\left[\frac{m_{A} m_{B}}{r_{A B}}+\frac{m_{B} m_{C}}{r_{B C}}+\frac{m_{C} m_{A}}{r_{C A}}\right] $
$=6.7 \times 10^{-11}\left[\frac{350 \times 2000}{80 \times 10^{-2}}+\frac{2000 \times 500}{120 \times 10^{-2}}+\frac{350 \times 500}{40 \times 10^{-2}}\right] $
$=-6.7 \times 10^{-11}[8750+8333.33+4375] \times 10^{+2}$
$=-6.7 \times 10^{-9} \times 21458.33$
$=-143770.81 \times 10^{-9}$
$=-1.43 \times 10^{-4} J$
Hence gravitational potential energy
$U=-1.43 \times 10^{-4} J$
and its value shall increase if $m_{B}$ removed.