Q.
Three particles each of mass $m$ are placed at the vertices of an equilateral triangle of side $r$ as shown in the figure. The magnitude of the gravitational force on any one particle due to others two is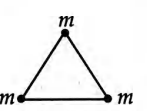
Gravitation
Solution:
