Question

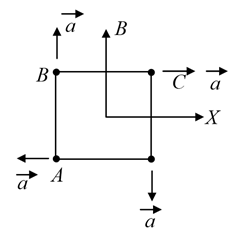
There are four particles $A,B,C$ and $D$ with masses $m_{A}=m,m_{B}=2\,m,m_{C}=3\,m$ and $m_{D}=4\,m$ are at the corners of a square. They have accelerations of equal magnitude with directions as shown. The acceleration of the center of mass of the particles is:

• A. $\frac{a}{5}\left(\right.\hat{i}-\hat{j}\left.\right)$
• B. $\frac{a}{5}\left(\right.\hat{i}+\hat{j}\left.\right)$
• C. $a\left(\right.\hat{i}+\hat{j}\left.\right)$
• D. Zero

Answer 1

Answer: (A)

$\therefore \overset{ \rightarrow }{a}_{cm}=\frac{m_{1} \overset{ \rightarrow }{a}_{1} + m_{2} \overset{ \rightarrow }{a}_{2} + \ldots }{m_{1} + m_{2} + \ldots }$
$=\frac{m \times \left(\right. - a \hat{i} \left.\right) + 2 m \left(\right. a \hat{j} \left.\right) + 3 m \left(\right. a \hat{i} \left.\right) + 4 m \left(\right. - a \hat{j} \left.\right)}{m + 2 m + 3 m + 4 m}=\left(\frac{2 \hat{i} - 2 \hat{j}}{10}\right)a=\frac{a}{5}\left(\right.\hat{i}-\hat{j}\left.\right)$