Q.
The wheel of radius $R$ rolls without slipping on horizontal rough surface, and its centre $O$ has an horizontal acceleration $a_{0}$ in forward direction. A point $P$ on the wheel is a distance $r$ from $O$ and angular position $\theta$ from horizontal. For the given values of $a_{0}, R$ and $r$, determine the angle $\theta$ for which point $P$ has no acceleration in this position.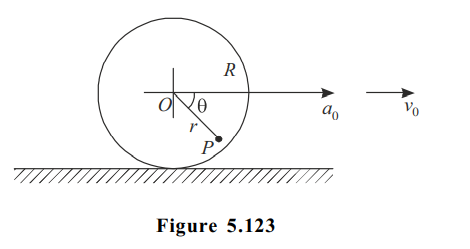
System of Particles and Rotational Motion
Solution:
