Question

The three processes in a thermodynamic cycle shown in the figure are :
Process $1\rightarrow 2$ is isothermal;
Process $2 \rightarrow 3$ is isochoric (volume remains constant);
Process $3 \rightarrow 1$ is adiabatic

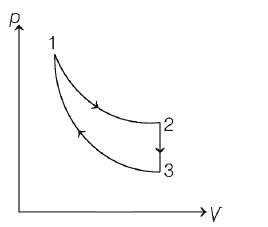
The total work done by the ideal gas in this cycle is $10\, J.$ The in ternal energy decreases by $20 \,J$ in the isochoric process. The work done by the gas in the adiabatic process is $-20\,J.$ The heat added to the system in the isothermal process is

• A. 0 J
• B. 10 J
• C. 20 J
• D. 30 J

Answer 1

Answer: (D)

Work done in complete cycle
$W=W_{12} +W_{23} +W_{31} ....(i)$

Given $W = 10\, J, W_{23} = 0$
and $W_{31} = - 20 \,J$
So, from Eq. (i), we have $W_{12} = 30 \,J$
As in isothermal process,
Heat absorbed = Work done
$\therefore $ Heat absorbed in process $1\rightarrow 2 = 30\, J$