Q.
The situation shown in the figure, all surfaces are frictionless. Masses of blocks $B$ and $C$ are $1\, kg$ and $2 kg$, respectively. Find the mass of the blocks $A$ for which the block $B$ remains stationary with respect to block $C$.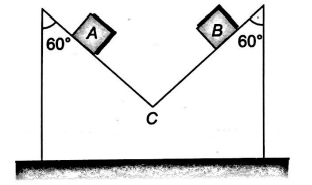
Laws of Motion
Solution:
