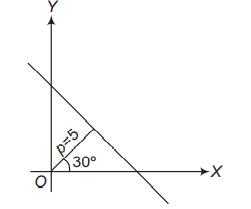
Question

The perpendicular distance between the line and origin is 5 units and the angle made by the perpendicular with the positive $X$-axis is $30^{\circ}$. Then, the equation of line is

• A. $x+\sqrt{3} y=10$
• B. $\sqrt{3} x+y=10$
• C. $x-\sqrt{3} y=10$
• D. $\sqrt{3} x-y=10$

Answer 1

Answer: (B)

Here, $p=5$ and $\alpha=30^{\circ}$

So, equation of line in normal form is
$x \cos \alpha+y \sin \alpha =p $
$\therefore x \cos 30^{\circ}+y \sin 30^{\circ} =5 $
$\Rightarrow \frac{\sqrt{3}}{2} x+\frac{1}{2} y=5 $
$ \Rightarrow \sqrt{3} x+y=10$