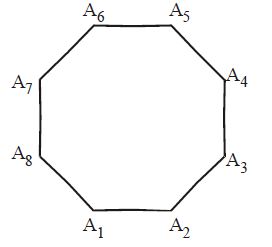
Q. The number of triangles whose vertices are at the vertices of an octagon but none of whose side happen to come from the sides of the octagon is
Permutations and Combinations
Solution:
Number of all possible triangles
= Number of selections of 3 points from 8 vertices
$=^8C_3=56$
Number of triangle with one side common with octagon $= 8 × 4 = 32$
(Consider side $A_1A_2$. Since two points $A_3, A_8$ are adjacent, 3rd point should be chosen from remaining 4 points.)
Number of triangles having two sides common with octagon : All such triangles have three consecutive vertices, viz., $A_1A_2A_3, A_2A_3A_4, ..... A_8A_1A_2.$
Number of such triangles $= 8$
$\therefore $ Number of triangles with no side common $= 56 - 32 - 8 = 16.$