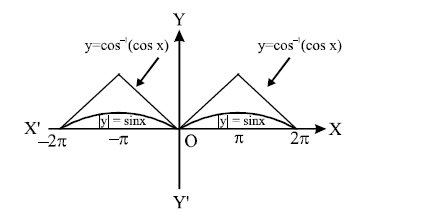
Q. The number of real solutions $( x , y )$ where $| y |=\sin x$, $y=\cos ^{-1}(\cos x),-2 \pi \leq x \leq 2 \pi$, is
Inverse Trigonometric Functions
Solution:
Graph of $|y|=\sin x$ and $y=\cos ^{-1}(\cos x)$ are shown in fig. It is evident from the graph that the curves intersect at $(0,0),(2 \pi, 0)$ and $(-2 \pi, 0)$.
Hence, there are $3$ solutions.