Q.
The force vs time graph of an object of mass $1\,kg$ which is initially at rest is shown below. What will be the graph of momentum vs time for the given graph?
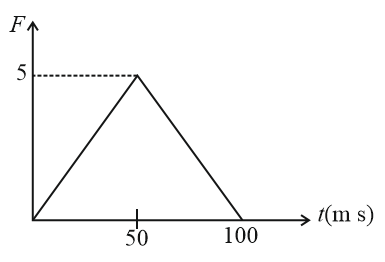
NTA AbhyasNTA Abhyas 2022
Solution:
