Q.
The following figure depict a circular motion. The radius of the circle, the period of revolution, the initial position and the sense of revolution are indicated on the figure. The simple harmonic motion of the $x$ - projection of the radius vector of the rotating particle $P$ can be shown as: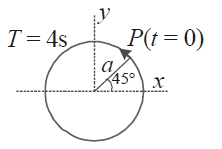
BITSATBITSAT 2017
Solution: