Question

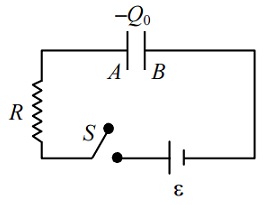
The figure shows an $RC$ circuit with a parallel plate capacitor. Before switching on the circuit, plate $A$ of the capacitor has a charge $-Q_{0}$ while plate $B$ has no net charge. If at $t=0$ , the switch is closed then after how much time (in seconds) will the net charge on plate A becomes zero?[Given: $C=1μF$ , $Q_{0}=1mC$ , $\epsilon =1000 \, V$ and $R=\frac{2 \times 10^{6}}{ln 3}\Omega$ ]

Answer 1

Let at any time $t$ the charge that flows from the plate $B$ to plate $A$ is $q$ and the instantaneous current is $I$ .

$\left(\frac{2 q - Q_{0}}{2 C}\right)+IR-\epsilon =0$
$\Rightarrow R\frac{d q}{d t}=\frac{- 2 q + 2 \epsilon C + Q_{0}}{2 C}$
$\Rightarrow \frac{d q}{2 \epsilon C + Q_{0} - 2 q}=\frac{d t}{2 R C}$
Now for the charge on plate $A$ to be zero $q=Q_{0}$ .
$\displaystyle \int _{0}^{Q_{0}}\frac{d q}{2 \epsilon C + Q_{0} - 2 q}=\displaystyle \int _{0}^{t}\frac{d t}{2 R C}$
$\Rightarrow t=RCln\left[\frac{2 \epsilon C + Q_{0}}{2 \epsilon C - Q_{0}}\right]=2s$