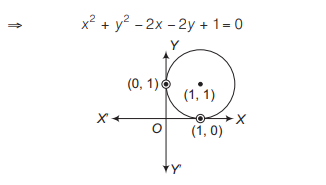
Question

The equation of the circle in the first quadrant touching each coordinate axis at a distance of one unit from the origin is

• A. $x^2+y^2-2 x-2 y+1=0$
• B. $x^2+y^2-2 x-2 y-1=0$
• C. $x^2+y^2-2 x-2 y=0$
• D. $x^2+y^2-2 x+2 y-1=0$

Answer 1

Answer: (A)

(Since, the circle is in the first quadrant touching each co-ordinate axis at a distance of one unit from origin.
$\therefore$ It passes through the points $(1,0)$ and $(0,1)$.
Also, its centre will be $(1,1)$ and radius is one unit. Hence, the equation of required circle is
$(x-1)^2+(y-1)^2=1^2$