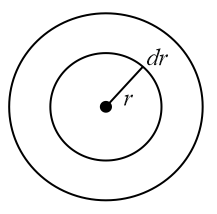
Question

The electric potential in a region is given as $V=-4ar^{2}+3b$ , where $r$ is distance from the origin, $a \, $ and $b$ are constants. If the volume charge density in the region is given by $\rho =na\epsilon _{0}$ , then what is the value of $n$ ?

Answer 1

$V=-4ar^{2}+3b$
$E=\frac{- d V}{ d r}=8ar$
The small amount of flux $d\phi$ associated with the closed surface bounded by two spheres of radius $r$ and $r+dr$ is
$d\phi=\left(E + d E\right)\left(A + d A\right)-EA$
$d\phi=EdA+dEA$
$dE=8adr$ and $dA=8\pi rdr$
Using Gauss's law
$\frac{\rho \times 4 \pi r^{2} d r}{\epsilon _{0}}=8adr\times 4\pi r^{2}+8ar\times 8\pi rdr$
⇒ $\rho =24a\epsilon _{0}$
$n=24$