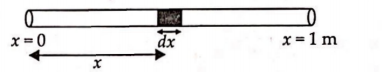
Question

The density of a non-uniform rod of length $1 \,m$ is given by $\rho(x) = a( 1 + bx^2)$ where a and b are constants and $0 \le x \le 1$. The centre of mass of the rod will be at

• A. $\frac{3\left(2+b\right)}{4\left(3+b\right)}$
• B. $\frac{4\left(2+b\right)}{3\left(3+b\right)}$
• C. $\frac{3\left(3+b\right)}{4\left(2+b\right)}$
• D. $\frac{4\left(3+b\right)}{3\left(2+b\right)}$

Answer 1

Answer: (A)

Mass of a small element of length $dx$ of the rod at a distance $x$ from the one end of the rod is
$dm = \rho dx = a( 1 + bx^2)dx$
The centre of mass of the rod is
$X_{CM } =\frac{ \int\limits_{0}^{1} x dm }{\int\limits_{0}^{1} dm } = \frac{ \int\limits_{0}^{1} xa \left(1+bx^{2}\right)dx}{ \int\limits_{0}^{1}a\left(1+bx^{2}\right)dx} $
$=\frac{ \int\limits_{0}^{1}\left(x+bx^{3}dx\right)}{ \int\limits_{0}^{1}\left(1+bx^{2}\right)dx} = \frac{\left[\frac{x^{2}}{2} +\frac{bx^{4}}{4}\right]_{0}^{1}}{\left[x+\frac{bx^{3}}{3}\right]_{0}^{1}}$
$ = \frac{\left[\frac{1}{2}+\frac{b}{4}\right]}{\left[1+\frac{b}{3}\right]} = \frac{3\left(2+b\right)}{4\left(3+b\right)}$