Q.
The adjoining figure shows a current carrying loop where the arc $PQRS$ is a three quarter circle and $PS$ is a straight line segment. A uniform magnetic field $B$ exists throughout the region with its direction being along the $-z$ axis. If it is given that the force on branch $PS$ is $F$ , then calculate the force on branch $PQR$ in terms of $F$ .
NTA AbhyasNTA Abhyas 2020
Solution:
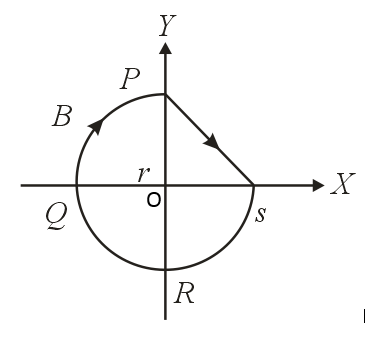
$POS$ is a right angled triangle. where
$\left(PS\right)^{2}=\left(PO\right)^{2}+\left(OS\right)^{2}$
$\Rightarrow PS=\sqrt{r^{2} + r^{2}}=\sqrt{2}r$
$\therefore $ Length of $PS$ will be equal to
$PS=\sqrt{2}r$
$F_{P S}=ilB=i\sqrt{2}rB=F\left(\right.Given\left.\right)$
Consider the vector length of the semicircular arc $PQR$ which is equal to the length $PR$ .
$PQR=2r$
$F_{P Q R}=i\left(\right.PR\left.\right)B$
$\Rightarrow F_{P Q R}=i.2r.B$
$\Rightarrow F_{P Q R}=2irB$
$\Rightarrow F_{P Q R}=\sqrt{2}F\left[\because F = \sqrt{2} i r B\right]$
$POS$ is a right angled triangle. where
$\left(PS\right)^{2}=\left(PO\right)^{2}+\left(OS\right)^{2}$
$\Rightarrow PS=\sqrt{r^{2} + r^{2}}=\sqrt{2}r$
$\therefore $ Length of $PS$ will be equal to
$PS=\sqrt{2}r$
$F_{P S}=ilB=i\sqrt{2}rB=F\left(\right.Given\left.\right)$
Consider the vector length of the semicircular arc $PQR$ which is equal to the length $PR$ .
$PQR=2r$
$F_{P Q R}=i\left(\right.PR\left.\right)B$
$\Rightarrow F_{P Q R}=i.2r.B$
$\Rightarrow F_{P Q R}=2irB$
$\Rightarrow F_{P Q R}=\sqrt{2}F\left[\because F = \sqrt{2} i r B\right]$