Q.
Six resistors of $3 \Omega$ each are connected along the sides of a hexagon and three resistors of $6 \Omega$ each are connected along $A C, A D$ and $A E$ as shown in the figure. The equivalent resistance between $A$ and $B$ is equal to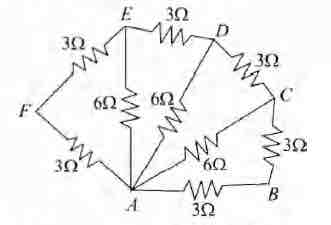
AIPMTAIPMT 1994Current Electricity
Solution:
