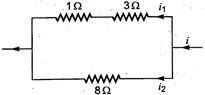
Question

Power dissipated across the $8 \, \Omega $ resistor in the circuit shown here is $2W$. The power dissipated in watt units across the $3 \, \Omega $ resistor is

• A. 2
• B. 1
• C. 0.5
• D. 3

Answer 1

Answer: (D)

Resistances $1 \,\Omega$ and $3 \,\Omega$ are connected in series,
so effective resistance $R=1+3=4\, \Omega$
Now, $R$ and $80$ are in parallel.
We know that potential difference across resistances in parallel order is same
Hence, $R \times i_{1}=8 i_{2}$
or $4 \times i_{1}=8 i_{2}$
or $i_{1}=\frac{8}{4} i_{2}=2 i_{2}$
or $i_{1}=2 i_{2}$ ...(i)
Power dissipated across $8\, \Omega$ resistance is
$i_{2}^{2}(8) t=2 W$
or $i_{2}^{2} t=\frac{2}{8}=0.25 \,W$ ...(ii)
Power dissipated across $3 \,\Omega$ resistance is
$H=i_{1}^{2}(3) t=\left(2 i_{2}\right)^{2}(3) t$
$=12 i_{2}^{2} t$
but $i_{2}^{2} t=0.25\, W $
$\therefore H=12 \times 0.25=3 \,W$