Q.
One plate of a parallel plate capacitor is connected to a spring as shown in the figure. The area of each plate of the capacitor is $A$ and the distance between the plates is $d$, when the battery is not connected and the spring is unstretched. After connecting the battery, in the steady state the distance between the plates is $0.75\, d$, then the force constant of the spring is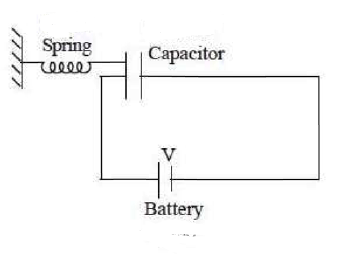
AP EAMCETAP EAMCET 2018
Solution: