Question

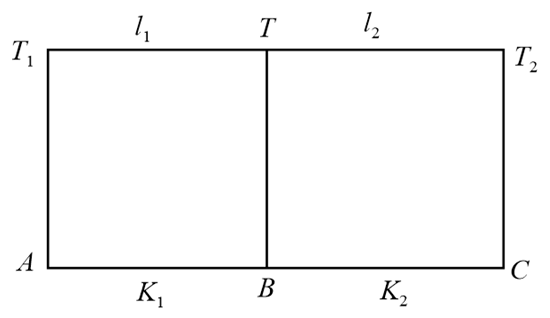
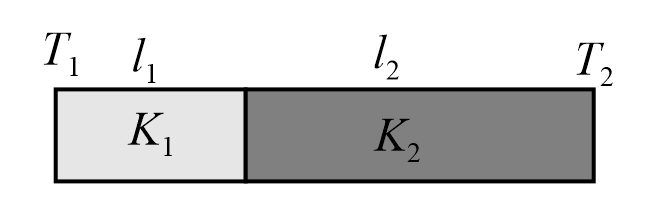
One end of a thermally insulated rod is kept at a temperature $T_{1}$ and other at $T_{2}$ . The rod is composed of two sections of lengths $l_{1}$ and $l_{2}$ and thermal conductivities $K_{1}$ and $K_{2}$ respectively. The temperature at the interface of the two sections is

• A. $\frac{\left(\left(\right. K\right)_{2} l_{2} T_{1} + K_{1} l_{1} T_{2} \left.\right)}{\left(\right. K_{1} l_{1} + K_{2} l_{2} \left.\right)}$
• B. $\frac{\left(\left(\right. K\right)_{2}l_{1}T_{1}+K_{1}l_{2}T_{2}\left.\right)}{\left(\right. K_{2} l_{1} + K_{1} l_{2} \left.\right)}$
• C. $\frac{\left(\left(\right. K\right)_{1}l_{2}T_{1}+K_{2}l_{1}T_{2}\left.\right)}{\left(\right. K_{1} l_{2} + K_{2} l_{1} \left.\right)}$
• D. $\frac{\left(\left(\right. K\right)_{1}l_{1}T_{1}+K_{2}l_{2}T_{2}\left.\right)}{\left(\right. K_{1} l_{1} + K_{2} l_{2} \left.\right)}$

Answer 1

Answer: (C)

Let temperature at the interface is $T$ .
For part $AB$ ,

$\frac{Q_{1}}{t} \propto \frac{\left(T_{1} - T\right) K_{1}}{l_{1}}$
For part $BC$ ,
$\frac{Q_{2}}{t} \propto \frac{\left(T - T_{2}\right) K_{2}}{l_{2}}$
At equilibrium, $\frac{Q_{1}}{t}=\frac{Q_{2}}{t}$
$\therefore $ $\frac{\left(T_{1} - T\right) K_{1}}{l_{1}}=\frac{\left(T - T_{2}\right) K_{2}}{l_{2}}$
$\Rightarrow $ $ \, T=\frac{T_{1} K_{1} l_{2} + T_{2} K_{2} l_{1}}{K_{1} l_{2} + K_{2} l_{1}}$