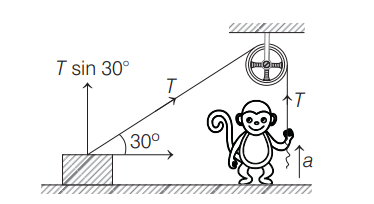
Q.
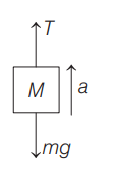
One end of a light string is fixed to a clamp on the ground and the other end passes over a fixed frictionless pulley as shown in the figure. It makes an angle of $30^{\circ}$ with the ground. The clamp can tolerate a vertical force of $40 \,N$. If a monkey of mass $5 \,kg$ were to climb up the rope, then the maximum acceleration in the upward direction with which it can climb safely is $\left(g=10\, ms ^{-2}\right)$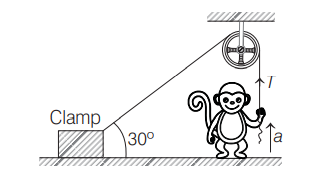
AP EAMCETAP EAMCET 2018
Solution: